 |
BUILT-IN MATHEMATICA SYMBOL
Divisible
Details
- Divisible[n, m] works for integers or rational numbers n and m.
- Divisible works with exact numeric quantities, as well as explicit numbers.
- Divisible works with exact complex numbers.
- Divisible[n, m] yields True only if
is an integer. - Divisible[n, m] is effectively equivalent to Mod[n, m]==0.
- For exact numeric quantities, Divisible internally uses numerical approximations to establish its result. This process can be affected by the setting of the global variable $MaxExtraPrecision.
- Divisible[n, m] can be entered as
. 
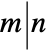can be entered as \[Divides] or EscdividesEsc.
Examplesopen all
Basic Examples (1)
Test whether 10 is divisible by 3:
| In[1]:= |
| Out[1]= |  |
New in 6
