HurwitzLerchPhi[z,s,a]
gives the Hurwitz–Lerch transcendent ![]() .
.


HurwitzLerchPhi
HurwitzLerchPhi[z,s,a]
gives the Hurwitz–Lerch transcendent ![]() .
.
Details

- Mathematical function, suitable for both symbolic and numerical manipulation.
- The Hurwitz–Lerch transcendent is defined as an analytic continuation of
![TemplateBox[{z, s, a}, HurwitzLerchPhi]=sum_(k=0)^(infty)z^k(k+a)^(-s) TemplateBox[{z, s, a}, HurwitzLerchPhi]=sum_(k=0)^(infty)z^k(k+a)^(-s)](Files/HurwitzLerchPhi.en/2.png) .
. - HurwitzLerchPhi is identical to LerchPhi for
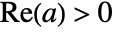 .
. - Unlike LerchPhi, HurwitzLerchPhi has singularities at
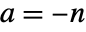 for non-negative integers
for non-negative integers 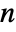 .
. - HurwitzLerchPhi has branch cut discontinuities in the complex
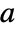 plane running from
plane running from 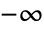 to
to 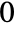 , and in the complex
, and in the complex 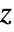 plane running from
plane running from 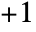 to
to 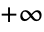 .
. - For certain special arguments, HurwitzLerchPhi automatically evaluates to exact values.
- HurwitzLerchPhi can be evaluated to arbitrary numerical precision.
- HurwitzLerchPhi automatically threads over lists.
- HurwitzLerchPhi can be used with Interval and CenteredInterval objects. »
Examples
open all close allBasic Examples (7)
Simple exact values are generated automatically:
Plot over a subset of the reals:
Plot over a subset of the complexes:
Series expansion at the origin:
Series expansion at Infinity:
Scope (29)
Numerical Evaluation (6)
The precision of the output tracks the precision of the input:
Evaluate efficiently at high precision:
Compute worst-case guaranteed intervals using Interval and CenteredInterval objects:
Compute average-case statistical intervals using Around:
Compute the elementwise values of an array:
Or compute the matrix HurwitzLerchPhi function using MatrixFunction:
Specific Values (5)
Simple exact values are generated automatically:
HurwitzLerchPhi[z,s,a] for symbolic a:
HurwitzLerchPhi[z,s,a] for symbolic z:
Find a value of z for which HurwitzLerchPhi[z,1,1/2]=2.5:
Visualization (2)
Plot the HurwitzLerchPhi function:
Plot the real part of the HurwitzLerchPhi function:
Plot the imaginary part of the HurwitzLerchPhi function:
Function Properties (12)
Real domain of HurwitzLerchPhi:
The defining sum for HurwitzLerchPhi:
HurwitzLerchPhi threads elementwise over lists:
![]() is neither non-decreasing nor non-increasing:
is neither non-decreasing nor non-increasing:
![]() is neither non-negative nor non-positive:
is neither non-negative nor non-positive:
![]() has both singularity and discontinuity for x0 or for x≥1:
has both singularity and discontinuity for x0 or for x≥1:
![]() is neither convex nor concave:
is neither convex nor concave:
TraditionalForm formatting:
Differentiation (3)
Series Expansions (1)
Find the Taylor expansion using Series:
Applications (1)
The moments and central moments of the geometric distribution can be expressed using HurwitzLerchPhi:
Properties & Relations (2)
Some hypergeometric functions can be expressed in terms of HurwitzLerchPhi:
Sum can generate HurwitzLerchPhi:
Possible Issues (2)
HurwitzLerchPhi differs from LerchPhi by a different choice of branch cut:
HurwitzLerchPhi includes singular terms, unlike LerchPhi:
See Also
Related Guides
Text
Wolfram Research (2008), HurwitzLerchPhi, Wolfram Language function, https://reference.wolfram.com/language/ref/HurwitzLerchPhi.html (updated 2023).
CMS
Wolfram Language. 2008. "HurwitzLerchPhi." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2023. https://reference.wolfram.com/language/ref/HurwitzLerchPhi.html.
APA
Wolfram Language. (2008). HurwitzLerchPhi. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/HurwitzLerchPhi.html
BibTeX
@misc{reference.wolfram_2025_hurwitzlerchphi, author="Wolfram Research", title="{HurwitzLerchPhi}", year="2023", howpublished="\url{https://reference.wolfram.com/language/ref/HurwitzLerchPhi.html}", note=[Accessed: 05-September-2025]}
BibLaTeX
@online{reference.wolfram_2025_hurwitzlerchphi, organization={Wolfram Research}, title={HurwitzLerchPhi}, year={2023}, url={https://reference.wolfram.com/language/ref/HurwitzLerchPhi.html}, note=[Accessed: 05-September-2025]}