DOCUMENTATION CENTER SEARCH

New to Mathematica? Find your learning path »

Mathematica > Core Language > List Manipulation > Rearranging & Restructuring Lists > ArrayFlatten >

Mathematica > Data Manipulation > Handling Arrays of Data > Rearranging & Restructuring Lists > ArrayFlatten >

Mathematica > Data Manipulation > Image Processing & Analysis > Basic Image Manipulation > Handling Arrays of Data > Rearranging & Restructuring Lists > ArrayFlatten >

ArrayFlatten

creates a single flattened matrix from a matrix of matrices

.

flattens out r pairs of levels in the array a.

MORE INFORMATION

ArrayFlatten requires that the blocks it flattens have dimensions that fit together.

ArrayFlatten can be used to form block matrices from arrays of blocks.

For a matrix of matrices, ArrayFlatten[a] yields a matrix whose elements are in the same order as in MatrixForm[a].

ArrayFlatten[a] is normally equivalent to Flatten. »

ArrayFlatten is normally equivalent to Flatten.

For a tensor with rank 2r, ArrayFlatten gives a tensor with rank r.

In ArrayFlatten all the matrices in the same row must have the same first dimension, and matrices in the same column must have the same second dimension.

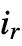In general, in ArrayFlatten, all the k dimensions of must be equal for each possible value of .

Elements at level r whose array depth is less than r are treated as scalars, and are replicated to fill out a rank r array of the appropriate dimensions.

ArrayFlatten works with SparseArray objects. »

Basic Examples (2)

Create a block matrix by flattening out a matrix of matrices:

Use 0s to represent zero matrices:

Create a block matrix by flattening out a matrix of matrices:

Out[2]=

Use 0s to represent zero matrices:

Out[2]=

Flatten a rank 4 array to rank 2:

Flatten only the first four levels of a rank 6 array:

Flatten a rank 6 array to rank 3:

ArrayFlatten works with SparseArray objects:

Make a sparse matrix from a block matrix of SparseArray objects:

Applications (4)

Put together many copies of a "tile":

Iterate a 2D substitution system:

Iterate a 3D substitution system:

Form a block matrix semidiscretization of the wave equation

with n spatial points:

Differentiation matrix for second-order approximation of

with periodic boundary conditions:

Identity matrix of size n:

Form block matrix a for system

where

:

Set up an initial condition vector for

:

Approximate the solution at

using the backward Euler method with time step k:

Show

and

at

:

Properties & Relations (3)

MatrixForm displays matrices of matrices in the same order as ArrayFlatten:

ArrayFlatten is a special case of Flatten:

KroneckerProduct is defined as ArrayFlatten of an Outer product:

Flatten Join Band ArrayQ ArrayDepth ArrayPad ImageApply

Rearranging Nested Lists

Combining Lists

Structural Operations

Vectors and Matrices

Tensors

New in 6

Ask a question about this page | Suggest an improvement | Leave a message for the team

Format: HTML | CDF