BUILT-IN MATHEMATICA SYMBOL

DegreeCentrality

DegreeCentrality[g]
gives a list of vertex degrees for the vertices in the underlying simple graph of g.

DegreeCentrality

gives a list of vertex in-degrees.

DegreeCentrality

gives a list of vertex out-degrees.

MORE INFORMATION

The vertex degree for a vertex 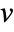v is the number of edges incident to v.

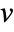For a directed graph, the in-degree is the number of incoming edges and the out-degree is the number of outgoing edges.

For an undirected graph, in-degree and out-degree coincide.

EXAMPLES

CLOSE ALL

Basic Examples (2)

Find the degree for each vertex:

Find the in-degree and out-degree for each vertex:

Find the degree for each vertex:

In[1]:=

Out[1]=

Find the in-degree and out-degree for each vertex:

In[1]:=

In[2]:=

Out[2]=

In[3]:=

Out[3]=

Scope (3)

DegreeCentrality for undirected graphs:

Directed graphs:

In-degree and out-degree:

Works with large graphs:

Applications (4)

Highlight the degree centrality for CycleGraph:

An unbalanced tree:

Label the vertex with in-degree and out-degree:

The degree is the sum of in- and out-degrees:

Vertex degrees of the HararyGraph:

Highlight and label the vertex by its degree:

Create a social network:

Find the people with more influence:

Properties & Relations (8)

The degree of a vertex of an undirected graph is the number of edges incident to the vertex:

For an undirected graph, the vertex in-degree and out-degree are equal to the vertex degree:

For a directed graph, the sum of the vertex in-degree and out-degree is the vertex degree:

Put the vertex degree, in-degree, and out-degree before, above, and below the vertex, respectively:

The sum of the degrees of all vertices of a graph is twice the number of edges:

Every graph has an even number of vertices with odd degree:

Each vertex of a

-regular graph has the same vertex degree

:

All vertices of a simple graph have a maximum degree less than the number of vertices:

A simple graph without isolated vertices has at least one pair of vertices with equal degrees: