 |
BUILT-IN MATHEMATICA SYMBOL
LinearFractionalTransform
LinearFractionalTransform[m]
gives a TransformationFunction that represents a linear fractional transformation defined by the homogeneous matrix m.
LinearFractionalTransform[{a, b, c, d}]
represents a linear fractional transformation that maps 
to 
.
Details
- LinearFractionalTransform gives a TransformationFunction that can be applied to vectors.
- For ordinary linear fractional transforms in n dimensions, m is an
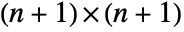matrix. - LinearFractionalTransform in general supports
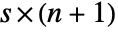matrices for transformations in 
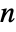dimensions. - In LinearFractionalTransform[{a, b, c, d}], a is a matrix, b and c are vectors, and d is a scalar.
New in 6
