BUILT-IN MATHEMATICA SYMBOL
PermutationReplace
PermutationReplace[expr, perm]
replaces each part in expr by its image under the permutation perm.
PermutationReplace[expr, gr]
returns the list of images of expr under all elements of the permutation group gr.
- For an integer
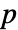in expr present in the cycles of the permutation perm, the image is the integer to the right of 
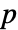, or the first integer of the cycle if 
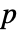is the last one. For an integer 
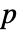not present in the cycles of perm, the image is 
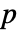itself.
- If g is a permutation object in expr, then the action is understood as right conjugation: PermutationProduct[InversePermutation[perm], g, perm]. This is equivalent to replacing the points in the cycles of g by their images under perm.
- When applied to a permutation group expr, PermutationReplace operates on each individual generator, returning the same abstract group but acting on different points.
- Both arguments are independently listable. If both arguments are lists then the second argument is threaded first.
The image of integer 4 under Cycles[{{2, 3, 4, 6}}] is integer 6:
| Out[1]= |  |
Under the identity, permutation integers are not moved:
| Out[2]= |  |
An action of a permutation on another permutation is understood as conjugation:
| Out[3]= |  |
Images under all elements of a group:
| Out[1]= |  |
New in 8
