ARMAProcess[{a1,…,ap},{b1,…,bq},v]
represents a weakly stationary autoregressive moving-average process with AR coefficients ai, MA coefficients bj, and normal white noise variance v.
ARMAProcess[{a1,…,ap},{b1,…,bq},Σ]
represents a weakly stationary vector ARMA process with coefficient matrices ai and bj and covariance matrix Σ.
ARMAProcess[{a1,…,ap},{b1,…,bq},v,init]
represents an ARMA process with initial data init.
ARMAProcess[c,…]
represents an ARMA process with a constant c.


ARMAProcess
ARMAProcess[{a1,…,ap},{b1,…,bq},v]
represents a weakly stationary autoregressive moving-average process with AR coefficients ai, MA coefficients bj, and normal white noise variance v.
ARMAProcess[{a1,…,ap},{b1,…,bq},Σ]
represents a weakly stationary vector ARMA process with coefficient matrices ai and bj and covariance matrix Σ.
ARMAProcess[{a1,…,ap},{b1,…,bq},v,init]
represents an ARMA process with initial data init.
ARMAProcess[c,…]
represents an ARMA process with a constant c.
Details


- ARMAProcess is also known as ARMA and VARMA (vector ARMA).
- ARMAProcess is a discrete-time and continuous‐state random process.
- The ARMA process is described by the difference equation
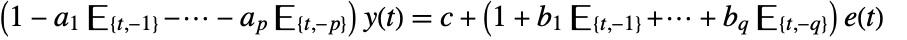 ,
where
,
where 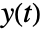 is the state output,
is the state output, 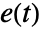 is white noise input,
is white noise input,  is the shift operator, and the constant c is taken to be zero if not specified.
is the shift operator, and the constant c is taken to be zero if not specified. - The initial data init can be given as a list {…,y[-2],y[-1]} or a single-path TemporalData object with time stamps understood as {…,-2,-1}.
- A scalar ARMA process should have real coefficients ai, bj, and c, and a positive variance v.
- An
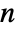 -dimensional vector ARMA process should have real coefficient matrices ai and bj of dimensions
-dimensional vector ARMA process should have real coefficient matrices ai and bj of dimensions 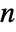 ×
×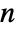 , real vector c of length n, and the covariance matrix Σ should be symmetric positive definite of dimensions
, real vector c of length n, and the covariance matrix Σ should be symmetric positive definite of dimensions 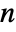 ×
×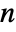 .
. - The ARMA process with zero constant has transfer function
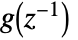 , where
, where 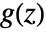 equals:
equals: -
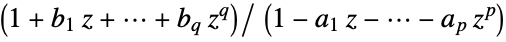
scalar process 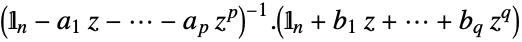
vector process; 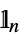 is the
is the 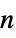 ×
×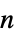 identity matrix
identity matrix - ARMAProcess[tproc,{p,q}] for a time series process tproc gives an ARMA process of orders p and q, such that its transfer function agrees with PadeApproximant about zero with degrees {q,p} of the transfer function of tproc.
- ARMAProcess[tproc] attempts to return an ARMA process such that its transfer function is the same as the one of tproc.
- Possible time series processes tproc include ARProcess, SARMAProcess, and SARIMAProcess.
- ARMAProcess[p,q] represents an ARMA process of orders p and q for use in EstimatedProcess and related functions.
- ARMAProcess can be used with such functions as CovarianceFunction, RandomFunction, and TimeSeriesForecast.
Examples
open all close allBasic Examples (3)
Scope (38)
Basic Uses (11)
Simulate an ensemble of paths:
Simulate with given precision:
Simulate a first-order scalar process:
Sample paths for positive and negative values of the parameter:
Simulate a weakly stationary process with given initial values:
For a process with a trend, initial values influence the behavior of the whole path:
Simulate a two-dimensional process:
Create a 2D sample path function from the data:
The color of the path is the function of time:
Create a 3D sample path function with time:
The color of the path is the function of time:
Simulate a three-dimensional process:
Create a sample path function from the data:
The color of the path is the function of time:
Compare the sample covariance functions with the one of the estimated process:
Use TimeSeriesModel to automatically find orders:
Compare the sample covariance functions with the best time series model:
Find maximum likelihood estimator:
Fix the constant and the variance and estimate the remaining parameters:
Plot the log-likelihood function together with the position of the estimated parameters:
Estimate a vector autoregressive moving average process:
Compare covariance functions for each component:
Find the forecast for the next 10 steps:
Plot the data and the forecasted values:
Find a forecast for a vector-valued time series process:
Covariance and Spectrum (6)
Closed-form correlation function for low order:
Covariance function for a vector-valued process:
Vector ARProcess:
Stationarity and Invertibility (5)
Estimation Methods (5)
The available methods for estimating an ARMAProcess:
Method of moments admits the following solvers:
This method allows for fixed parameters:
Some relations between parameters are also permitted:
Maximum conditional likelihood method allows the following solvers:
This method allows for fixed parameters:
Some relations between parameters are also permitted:
Maximum likelihood method allows the following solvers:
This method allows for fixed parameters:
Some relations between parameters are also permitted:
Spectral estimator allows users to specify windows used for PowerSpectralDensity calculation:
Spectral estimator allows the following solvers:
Process Slice Properties (5)
Single time SliceDistribution:
Multiple time slice distributions:
Slice distribution of a vector-valued time series:
First-order probability density function:
Compare with the density function of a normal distribution:
Compute the expectation of an expression:
Skewness and kurtosis are constant:
CentralMoment and its generating function:
FactorialMoment has no closed form for symbolic order:
Cumulant and its generating function:
Representations (6)
Approximate a SARMA process with an ARMAProcess[4,3]:
Compare the covariance function for the original and the approximate processes:
Approximate an ARIMA process with fixed initial conditions by an ARMA process:
Approximate a SARIMA process with fixed initial conditions with an ARMA process:
Represent an AR process as an equivalent ARMA process:
ARIMA with known integration order:
SARMA with known seasonal order:
SARIMA with known integration and seasonal orders:
TransferFunctionModel representation:
StateSpaceModel representation:
Applications (4)
Fit an ARMA model for the hourly measurements of temperature in August:
Check if the process is weakly stationary:
The low-order ARMA model does not capture the seasonal trend well:
Daily mean temperature readings in September 2012 near your location:
Check if the process is weakly stationary:
Plot forecast with original data:
The daily exchange rates of the euro to the dollar from May 2012 through September 2012:
Fit an ARMA process to the exchange rates:
Forecast for 10 business days ahead:
Plot forecast with original data:
Monthly water levels on Lake Mead:
Create TemporalData starting with observation from February 1935:
Check if the residuals exhibit any significant serial correlation:
Properties & Relations (7)
ARMAProcess is a generalization of MAProcess:
ARMAProcess is a generalization of ARProcess:
ARMAProcess is a special case of ARIMAProcess:
ARMAProcess is a special case of FARIMAProcess:
ARMAProcess is a special case of SARMAProcess:
ARMAProcess is a special case of SARIMAProcess:
Squared values of a GARCHProcess follow an ARMA process:
CorrelationFunction and PartialCorrelationFunction of squared values:
The corresponding ARMA process:
CorrelationFunction and PartialCorrelationFunction of the ARMA process:
Possible Issues (3)
Some properties are defined only for weakly stationary processes:
Use FindInstance to find a weakly stationary process:
ToInvertibleTimeSeries does not always exist:
There are zeros of the TransferFunctionModel on the unit circle:
The method of moments may not find a solution in estimation:
Neat Examples (2)
Simulate a weakly stationary three-dimensional ARMAProcess:
Non-weakly stationary process, starting at the origin:
Simulate paths from an ARMA process:
Take a slice at 50 and visualize its distribution:
Plot paths and histogram distribution of the slice distribution at 50:
Text
Wolfram Research (2012), ARMAProcess, Wolfram Language function, https://reference.wolfram.com/language/ref/ARMAProcess.html (updated 2014).
CMS
Wolfram Language. 2012. "ARMAProcess." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2014. https://reference.wolfram.com/language/ref/ARMAProcess.html.
APA
Wolfram Language. (2012). ARMAProcess. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/ARMAProcess.html
BibTeX
@misc{reference.wolfram_2025_armaprocess, author="Wolfram Research", title="{ARMAProcess}", year="2014", howpublished="\url{https://reference.wolfram.com/language/ref/ARMAProcess.html}", note=[Accessed: 26-October-2025]}
BibLaTeX
@online{reference.wolfram_2025_armaprocess, organization={Wolfram Research}, title={ARMAProcess}, year={2014}, url={https://reference.wolfram.com/language/ref/ARMAProcess.html}, note=[Accessed: 26-October-2025]}