represents the sporadic simple Conway group Co1.


ConwayGroupCo1
represents the sporadic simple Conway group Co1.
Background & Context
- ConwayGroupCo1[] represents the Conway group
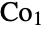 , which is a group of order
, which is a group of order ![TemplateBox[{2, 21}, Superscript].TemplateBox[{3, 9}, Superscript].TemplateBox[{5, 4}, Superscript].TemplateBox[{7, 2}, Superscript].11.13.23 TemplateBox[{2, 21}, Superscript].TemplateBox[{3, 9}, Superscript].TemplateBox[{5, 4}, Superscript].TemplateBox[{7, 2}, Superscript].11.13.23](Files/ConwayGroupCo1.en/2.png) . It is one of the 26 sporadic simple groups of finite order. Despite there being two other sporadic simple groups (ConwayGroupCo2 and ConwayGroupCo3) attributed to Conway, ConwayGroupCo1 is sometimes referred to as "the" Conway group.
. It is one of the 26 sporadic simple groups of finite order. Despite there being two other sporadic simple groups (ConwayGroupCo2 and ConwayGroupCo3) attributed to Conway, ConwayGroupCo1 is sometimes referred to as "the" Conway group. - The Conway group
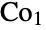 is the fifth largest of the sporadic finite simple groups. It was introduced by John Horton Conway in the late 1960s. ConwayGroupCo1 has a number of permutation representations, the smallest of which is a faithful on 98280 points. However, it is equivalently and most commonly defined as the quotient of the automorphism group of the so-called Leech lattice by its center. Along with the other sporadic simple groups, the Conway groups played a foundational role in the monumental (and complete) classification of finite simple groups.
is the fifth largest of the sporadic finite simple groups. It was introduced by John Horton Conway in the late 1960s. ConwayGroupCo1 has a number of permutation representations, the smallest of which is a faithful on 98280 points. However, it is equivalently and most commonly defined as the quotient of the automorphism group of the so-called Leech lattice by its center. Along with the other sporadic simple groups, the Conway groups played a foundational role in the monumental (and complete) classification of finite simple groups. - The usual group theoretic functions may be applied to ConwayGroupCo1[], including GroupOrder, GroupGenerators, GroupElements and so on. However, while ConwayGroupCo1[] is a permutation group, due its large order, an explicit permutation representation is impractical for direct implementation. As a result, a number of such group theoretic functions may return unevaluated when applied to it. A number of precomputed properties of the Conway group
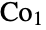 are available via FiniteGroupData[{"Conway",1},"prop"].
are available via FiniteGroupData[{"Conway",1},"prop"]. - ConwayGroupCo1 is related to a number of other symbols. ConwayGroupCo1 is one of the seven groups (along with ConwayGroupCo2, ConwayGroupCo3, JankoGroupJ2, HigmanSimsGroupHS, McLaughlinGroupMcL and SuzukiGroupSuz) collectively referred to as the "second generation" of sporadic finite simple groups. It is also one of 20 so-called "happy" sporadic groups, which all appear as a subquotient of the monster group.
See Also
Tech Notes
Related Guides
History
Text
Wolfram Research (2010), ConwayGroupCo1, Wolfram Language function, https://reference.wolfram.com/language/ref/ConwayGroupCo1.html.
CMS
Wolfram Language. 2010. "ConwayGroupCo1." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/ConwayGroupCo1.html.
APA
Wolfram Language. (2010). ConwayGroupCo1. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/ConwayGroupCo1.html
BibTeX
@misc{reference.wolfram_2025_conwaygroupco1, author="Wolfram Research", title="{ConwayGroupCo1}", year="2010", howpublished="\url{https://reference.wolfram.com/language/ref/ConwayGroupCo1.html}", note=[Accessed: 07-October-2025]}
BibLaTeX
@online{reference.wolfram_2025_conwaygroupco1, organization={Wolfram Research}, title={ConwayGroupCo1}, year={2010}, url={https://reference.wolfram.com/language/ref/ConwayGroupCo1.html}, note=[Accessed: 07-October-2025]}