ControllabilityMatrix
给出状态空间模型 ssm 的可控性矩阵.
更多信息

- 对于具有状态方程的标准状态空间模型:
-
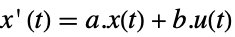
连续时间系统 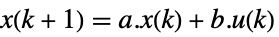
离散时间系统 - 可控性矩阵根据
 计算,其中
计算,其中  是
是  的维度.
的维度. - 对于具有状态方程的描述符状态空间模型:
-
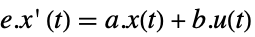
连续时间系统 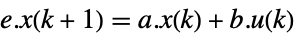
离散时间系统 - 慢速和快速子系统可以以 KroneckerModelDecomposition 中描述的方法解耦:
-

慢速子系统 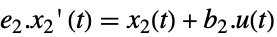
快速子系统 - ControllabilityMatrix 根据解耦型慢速和快速子系统返回矩阵对 {q1,q2}. 矩阵 q1 和 q2 定义如下,其中
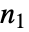 是
是 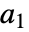 的维度,而
的维度,而  是
是  的幂零指数.
的幂零指数. -
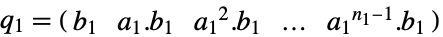
慢速子系统 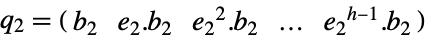
快速子系统 - 只有当描述符系统对于某个 λ 有 Det[λ e-a]≠0 成立时,可控性矩阵存在.
范例
打开所有单元关闭所有单元范围 (5)
Wolfram Research (2010),ControllabilityMatrix,Wolfram 语言函数,https://reference.wolfram.com/language/ref/ControllabilityMatrix.html (更新于 2012 年).
文本
Wolfram Research (2010),ControllabilityMatrix,Wolfram 语言函数,https://reference.wolfram.com/language/ref/ControllabilityMatrix.html (更新于 2012 年).
CMS
Wolfram 语言. 2010. "ControllabilityMatrix." Wolfram 语言与系统参考资料中心. Wolfram Research. 最新版本 2012. https://reference.wolfram.com/language/ref/ControllabilityMatrix.html.
APA
Wolfram 语言. (2010). ControllabilityMatrix. Wolfram 语言与系统参考资料中心. 追溯自 https://reference.wolfram.com/language/ref/ControllabilityMatrix.html 年