MathieuCharacteristicExponent
MathieuCharacteristicExponent[a,q]
给出具有特征值 a 和参数 q 的 Mathieu 函数的特征指数 r.
更多信息
- 数学函数,适宜于符号和数值运算.
- 所有 Mathieu 函数都具有
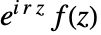 的形式,这里
的形式,这里 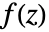 的周期为
的周期为 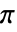 ,r是 Mathieu 特征指数.
,r是 Mathieu 特征指数. - 对某些特定参数,MathieuCharacteristicExponent 自动计算出精确值.
- MathieuCharacteristicExponent 可求任意数值精度的值.
- MathieuCharacteristicExponent 自动逐项作用于列表的各个元素.
范例
打开所有单元关闭所有单元范围 (15)
数值计算 (7)
MathieuCharacteristicExponent 按元素线性作用于列表:
用 Around 计算普通的统计区间:
或用 MatrixFunction 计算矩阵形式的 MathieuCharacteristicExponent 函数:
特殊值 (2)
可视化 (3)
绘制整数参数的 MathieuCharacteristicExponent 函数:
绘制非整数参数的 MathieuCharacteristicExponent 函数:
函数的属性 (3)
MathieuCharacteristicExponent[3,x] 既不是非递增,也不是非递减:
MathieuCharacteristicExponent[3,x] 既不是非负,也不是非正:
MathieuCharacteristicExponent[3,x] 既不凸,也不凹:
应用 (2)
根据 Bloch 定理,方程的解有界的前提是 ![]() 位于一个能带中. 能隙则对应于
位于一个能带中. 能隙则对应于 ![]() 的范围中 MathieuCharacteristicExponent 虚部不为零的部分:
的范围中 MathieuCharacteristicExponent 虚部不为零的部分:
属性和关系 (2)
文本
Wolfram Research (1996),MathieuCharacteristicExponent,Wolfram 语言函数,https://reference.wolfram.com/language/ref/MathieuCharacteristicExponent.html.
CMS
Wolfram 语言. 1996. "MathieuCharacteristicExponent." Wolfram 语言与系统参考资料中心. Wolfram Research. https://reference.wolfram.com/language/ref/MathieuCharacteristicExponent.html.
APA
Wolfram 语言. (1996). MathieuCharacteristicExponent. Wolfram 语言与系统参考资料中心. 追溯自 https://reference.wolfram.com/language/ref/MathieuCharacteristicExponent.html 年