MathieuCharacteristicB

MathieuCharacteristicB[r,q]

gives the characteristic value for odd Mathieu functions with characteristic exponent r and parameter q.

Details

Mathematical function, suitable for both symbolic and numerical manipulation.
The characteristic value 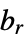gives the value of the parameter 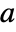in 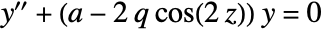for which the solution has the form 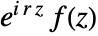where 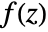is an odd function of with period .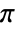
When r is not a real integer, MathieuCharacteristicB gives the same results as MathieuCharacteristicA.
For certain special arguments, MathieuCharacteristicB automatically evaluates to exact values.
MathieuCharacteristicB can be evaluated to arbitrary numerical precision.
MathieuCharacteristicB automatically threads over lists.

Examples

open allclose all

Basic Examples (3)

Evaluate numerically:

Plot over a subset of the reals:

Plot over a subset of the complexes:

Scope (15)

Numerical Evaluation (4)

Evaluate numerically:

Evaluate to high precision:

The precision of the output tracks the precision of the input:

Complex number inputs:

Evaluate efficiently at high precision:

Specific Values (2)

Simple exact values are generated automatically:

Find the positive maximum of MathieuCharacteristicB[3,q]:

Visualization (3)

Plot the MathieuCharacteristicB function for integer parameters:

Plot the MathieuCharacteristicB function for noninteger parameters:

Plot the real part of MathieuCharacteristicB:

Plot the imaginary part of MathieuCharacteristicB:

Function Properties (6)

The real domain of MathieuCharacteristicB:

is a continuous function of :

is neither non-increasing nor non-decreasing:

is not injective:

MathieuCharacteristicB threads elementwise over lists:

TraditionalForm formatting:

Applications (4)

Symmetric periodic solutions of the Mathieu differential equation:

This shows the stability diagram for the Mathieu equation:

As a function of the first argument, MathieuCharacteristicB is a piecewise continuous function (called bands and band gaps in solid-state physics):

Solve the Laplace equation in an ellipse using separation of variables:

This finds a zero:

This plots an eigenfunction. It vanishes at the ellipse boundary:

Possible Issues (1)

There is no zero-order MathieuCharacteristicB:

Neat Examples (1)

Branch points of the Mathieu characteristic along the imaginary q axis:

Top

Enable JavaScript to interact with content and submit forms on Wolfram websites. Learn how