SingularValues
Since Version 5.0 (released in 2003), SingularValues has been superseded by SingularValueList and SingularValueDecomposition.
SingularValues[m]
gives the singular value decomposition for a numerical matrix 
. The result is a list 
, where 
is the list of singular values, and 
can be written as ConjugateTranspose[u]. DiagonalMatrix[w].v.
Details and Options
- SingularValues[m,Tolerance->t] specifies that singular values smaller than t times the maximum singular value are to be removed.
- The default setting Tolerance->Automatic typically takes
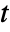to be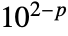 
where 
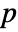is the numerical precision of the input. - With Tolerance->0 singular values which are exactly zero can be returned.
and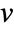 
are row orthonormal matrices, which can be considered as lists of orthonormal vectors.- The ratio of the largest to smallest singular value gives the
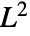condition number of 
.
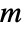Wolfram Research (1988), SingularValues, Wolfram Language function, https://reference.wolfram.com/language/ref/SingularValues.html.
Text
Wolfram Research (1988), SingularValues, Wolfram Language function, https://reference.wolfram.com/language/ref/SingularValues.html.
CMS
Wolfram Language. 1988. "SingularValues." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/SingularValues.html.
APA
Wolfram Language. (1988). SingularValues. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/SingularValues.html