ChebyshevT
ChebyshevT[n,x]
gives the Chebyshev polynomial of the first kind ![]() .
.
Details

- Mathematical function, suitable for both symbolic and numerical manipulation.
- Explicit polynomials are given for integer n.
![TemplateBox[{n, {cos, , theta}}, ChebyshevT]=cos(ntheta) TemplateBox[{n, {cos, , theta}}, ChebyshevT]=cos(ntheta)](Files/ChebyshevT.en/2.png) .
. - For certain special arguments, ChebyshevT automatically evaluates to exact values.
- ChebyshevT can be evaluated to arbitrary numerical precision.
- ChebyshevT automatically threads over lists.
- ChebyshevT[n,z] has a branch cut discontinuity in the complex z plane running from
 to
to 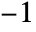 if n is not an integer.
if n is not an integer. - ChebyshevT can be used with Interval and CenteredInterval objects. »
Examples
open allclose allBasic Examples (7)
Compute the ![]() Chebyshev polynomial:
Chebyshev polynomial:
Plot over a subset of the reals:
Plot over a subset of the complexes:
Series expansion at the origin:
Asymptotic expansion at Infinity:
Scope (43)
Numerical Evaluation (5)
The precision of the output tracks the precision of the input:
Evaluate efficiently at high precision:
ChebyshevT can be used with Interval and CenteredInterval objects:
Specific Values (7)
Values of ChebyshevT at fixed points:
ChebyshevT for symbolic n:
Find the first positive maximum of ChebyshevT[5,x]:
Compute the associated ChebyshevT[7,x] polynomial:
Compute the associated ChebyshevT[1/2,x] polynomial for half-integer n:
Visualization (3)
Plot the ChebyshevT function for various orders:
Plot the Chebyshev polynomial as a function of two variables:
Function Properties (14)
ChebyshevT is defined for all real values from the interval [-1,∞]:
ChebyshevT is defined for all complex values:
![]() achieves all real and complex values:
achieves all real and complex values:
It achieves all complex values:
Chebyshev polynomial of an odd order is odd:
Chebyshev polynomial of an even order is even:
ChebyshevT threads elementwise over lists:
Chebyshev polynomials are analytic:
In general, ChebyshevT is neither analytic nor meromorphic:
![]() is neither non-decreasing nor non-increasing:
is neither non-decreasing nor non-increasing:
![]() is neither non-negative nor non-positive:
is neither non-negative nor non-positive:
![]() has singularities and discontinuities for
has singularities and discontinuities for ![]() when
when ![]() is not an integer:
is not an integer:
TraditionalForm formatting:
Differentiation (3)
Integration (4)
Compute the indefinite integral using Integrate:
Definite integral of ChebyshevT over a period for odd integers is 0:
Series Expansions (3)
Find the Taylor expansion using Series:
Plots of the first three approximations around ![]() :
:
General term in the series expansion using SeriesCoefficient:
Function Identities and Simplifications (4)
ChebyshevT is defined through the identity:
The ordinary generating function of ChebyshevT:
The exponential generating function of ChebyshevT:
Generalizations & Extensions (2)
Applications (4)
Plot the first 10 Chebyshev polynomials:
Find a minimax approximation to the function Clip[4 x]:
Get an expansion for a function in the Chebyshev polynomials:
The values of the function at the Chebyshev nodes:
Find the Chebyshev coefficients:
Solve a differential equation with the ChebyshevT function as the inhomogeneous part:
Properties & Relations (7)
Use FullSimplify with ChebyshevT:
Derivative of ChebyshevT is expressed in terms of ChebyshevU:
ChebyshevT can be represented as a DifferenceRoot:
General term in the series expansion of ChebyshevT:
The generating function for ChebyshevT:
The exponential generating function for ChebyshevT:
Possible Issues (1)
Text
Wolfram Research (1988), ChebyshevT, Wolfram Language function, https://reference.wolfram.com/language/ref/ChebyshevT.html (updated 2022).
CMS
Wolfram Language. 1988. "ChebyshevT." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2022. https://reference.wolfram.com/language/ref/ChebyshevT.html.
APA
Wolfram Language. (1988). ChebyshevT. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/ChebyshevT.html