SystemsModelStateFeedbackConnect[sys,con]
connects the states of the systems model sys to the controller con and the outputs of con to the inputs of sys in feedback.
SystemsModelStateFeedbackConnect[sys,con,{s1,…},{{in1,ftype1},…}]
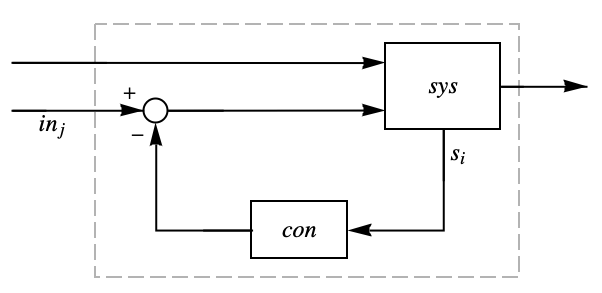
connects state si of sys to the i![]() input of con and the j
input of con and the j![]() output of con to input inj of sys with feedback type ftypej
output of con to input inj of sys with feedback type ftypej


SystemsModelStateFeedbackConnect
SystemsModelStateFeedbackConnect[sys,con]
connects the states of the systems model sys to the controller con and the outputs of con to the inputs of sys in feedback.
SystemsModelStateFeedbackConnect[sys,con,{s1,…},{{in1,ftype1},…}]
connects state si of sys to the i![]() input of con and the j
input of con and the j![]() output of con to input inj of sys with feedback type ftypej
output of con to input inj of sys with feedback type ftypej
Details

- The systems model sys can be a StateSpaceModel, AffineStateSpaceModel, or NonlinearStateSpaceModel.
- The controller con can be a gain matrix, a vector, StateSpaceModel, TransferFunctionModel, AffineStateSpaceModel, or NonlinearStateSpaceModel.
- StateFeedbackGains, LQRegulatorGains, DiscreteLQRegulatorGains, AsymptoticOutputTracker, and FullInformationOutputRegulator return state-feedback controllers as matrices or vectors.
Examples
open all close allBasic Examples (3)
Scope (15)
Basic Uses (10)
The closed-loop model of a system with state feedback:
The state feedback gains specified as a transfer-function model:
The gains specified as a state-space model:
A affine state-space model with feedback specified in a vector:
A nonlinear state-space model with feedback specified in a vector:
Only feedback to the first input:
Only feedback to the second input:
Connect the second state to the first input through a feedback system:
System Types (5)
Connect two StateSpaceModel systems:
Using descriptor state-space models:
Input linear AffineStateSpaceModel systems:
General nonlinear NonlinearStateSpaceModel systems:
Connecting a standard linear system and an input linear system will give an affine model:
Connecting standard linear or affine system with a nonlinear system gives a nonlinear model:
Applications (3)
The linearized inverted pendulum model is unstable:
A plot of its unstable response:
Compute a set of state feedback gains:
The closed-loop response is stable:
Calculate a gain matrix to optimize a quadratic cost function of the input and the states:
Calculate a gain matrix to optimize a quadratic cost function of the input and the outputs:
Properties & Relations (1)
A model where the output matrix is identity and the direct transmission matrix is zero:
SystemsModelStateFeedbackConnect and SystemsModelFeedbackConnect are equivalent:
Related Guides
Text
Wolfram Research (2010), SystemsModelStateFeedbackConnect, Wolfram Language function, https://reference.wolfram.com/language/ref/SystemsModelStateFeedbackConnect.html (updated 2014).
CMS
Wolfram Language. 2010. "SystemsModelStateFeedbackConnect." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2014. https://reference.wolfram.com/language/ref/SystemsModelStateFeedbackConnect.html.
APA
Wolfram Language. (2010). SystemsModelStateFeedbackConnect. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/SystemsModelStateFeedbackConnect.html
BibTeX
@misc{reference.wolfram_2025_systemsmodelstatefeedbackconnect, author="Wolfram Research", title="{SystemsModelStateFeedbackConnect}", year="2014", howpublished="\url{https://reference.wolfram.com/language/ref/SystemsModelStateFeedbackConnect.html}", note=[Accessed: 29-October-2025]}
BibLaTeX
@online{reference.wolfram_2025_systemsmodelstatefeedbackconnect, organization={Wolfram Research}, title={SystemsModelStateFeedbackConnect}, year={2014}, url={https://reference.wolfram.com/language/ref/SystemsModelStateFeedbackConnect.html}, note=[Accessed: 29-October-2025]}