DiscreteRiccatiSolve
DiscreteRiccatiSolve[{a,b},{q,r}]
gives the matrix ![]() that is the stabilizing solution of the discrete algebraic Riccati equation
that is the stabilizing solution of the discrete algebraic Riccati equation ![]() .
.
DiscreteRiccatiSolve[{a,b},{q,r,p}]
solves ![]() .
.
Details and Options

- In
![TemplateBox[{a}, ConjugateTranspose].x.a-x-TemplateBox[{a}, ConjugateTranspose].x.b.TemplateBox[{{(, {r, +, {TemplateBox[{b}, ConjugateTranspose], ., x, ., b}}, )}}, Inverse].TemplateBox[{b}, ConjugateTranspose].x.a+q=0 TemplateBox[{a}, ConjugateTranspose].x.a-x-TemplateBox[{a}, ConjugateTranspose].x.b.TemplateBox[{{(, {r, +, {TemplateBox[{b}, ConjugateTranspose], ., x, ., b}}, )}}, Inverse].TemplateBox[{b}, ConjugateTranspose].x.a+q=0](Files/DiscreteRiccatiSolve.en/4.png) ,
, 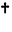 denotes the conjugate transpose.
denotes the conjugate transpose. - The equation
![TemplateBox[{a}, ConjugateTranspose].x.a-x-TemplateBox[{a}, ConjugateTranspose].x.b.TemplateBox[{{(, {r, +, {TemplateBox[{b}, ConjugateTranspose], ., x, ., b}}, )}}, Inverse].TemplateBox[{b}, ConjugateTranspose].x.a+q=0 TemplateBox[{a}, ConjugateTranspose].x.a-x-TemplateBox[{a}, ConjugateTranspose].x.b.TemplateBox[{{(, {r, +, {TemplateBox[{b}, ConjugateTranspose], ., x, ., b}}, )}}, Inverse].TemplateBox[{b}, ConjugateTranspose].x.a+q=0](Files/DiscreteRiccatiSolve.en/6.png) has a unique, symmetric, positive semidefinite solution only if
has a unique, symmetric, positive semidefinite solution only if 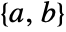 is stabilizable,
is stabilizable, 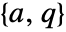 is detectable,
is detectable, 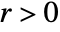 , and
, and 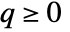 . Consequently, all the eigenvalues of the matrix
. Consequently, all the eigenvalues of the matrix 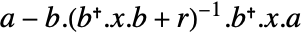 lie inside the unit circle, and the solution is stabilizing.
lie inside the unit circle, and the solution is stabilizing. - The solution is positive definite when
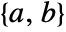 is controllable and
is controllable and 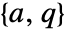 is observable.
is observable. - DiscreteRiccatiSolve supports a Method option. The following settings can be specified:
-
Automatic automatically determined method "Eigensystem" based on eigen decomposition "GeneralizedEigensystem" based on generalized eigen decomposition "GeneralizedSchur" based on generalized Schur decomposition "InverseFree" a variant of "GeneralizedSchur" "MatrixSign" - iterative method using the matrix sign function
"Newton" iterative Newton method "Schur" based on Schur decomposition - All methods apply to approximate numeric matrices. "Eigensystem" applies to exact matrices.
Examples
open allclose allScope (3)
Options (7)
Method (7)
Automatic and "Eigensystem" methods can be used for exact systems:
As well as for inexact systems:
"Schur" method can be used for inexact systems:
"Newton" applies to inexact systems and may be more accurate than Automatic:
Compute the solution and absolute error:
Compare with the default method:
"Newton" takes suboptions "StartingMatrix", "MaxIterations", and "Tolerance":
The "Newton" method may not compute a stabilizing solution even if such a solution exists:
Compare with the Automatic method:
"MatrixSign" is typically used as an initial approximation for the "Newton" method:
Use xinit to initialize the "Newton" method:
"MatrixSign" takes suboptions "MaxIterations" and "Tolerance":
"GeneralizedSchur" or "GeneralizedEigensystem" applies when a is singular:
These two methods work for a singular a:
"Eigensystem" cannot be used when a is singular:
"InverseFree" can be used when r is ill-conditioned:
The matrix r has a high condition number:
Compare to the default method:
The absolute error is higher for the default method in this case:
Applications (2)
The linearized model of a turbo-generator has state and input matrices:
The discrete LQ regulation cost Jopt for given weighting matrices {q,r} and initial state s0:
Compute an optimal state-feedback gain that guarantees that all the closed-loop poles are inside a circle of radius ![]() :
:
The closed-loop poles without any prescribed degree of stability:
Properties & Relations (11)
DiscreteRiccatiSolve[{a,b},{q,r,p}] is equivalent to DiscreteRiccatiSolve[{a-b.![]() .p,b},{q-p.r -1.p,r}]:
.p,b},{q-p.r -1.p,r}]:
An equivalent result can be found by incorporating p into the a and q matrices:
If {a,b} is stabilizable and {a,g} is detectable, and q=Transpose[g].g, then the solution to the discrete Riccati equation is positive semidefinite:
If {a,b} is controllable and {a,g} is observable, and q=Transpose[g].g, then the solution to the discrete Riccati equation is positive definite:
The matrix associated with the discrete algebraic Riccati equation is symplectic:
The eigenvalues of the symplectic matrix are pairs of the form ![]() :
:
The symplectic matrix must satisfy the stability and complementarity properties to obtain a stabilizing solution to the Riccati equation:
The eigenvalues of the feedback system with ![]() are the stable eigenvalues of the symplectic matrix:
are the stable eigenvalues of the symplectic matrix:
Compute optimal state-feedback gains using DiscreteRiccatiSolve:
Use LQRegulatorGains to obtain the same result directly:
Compute optimal output feedback gains using DiscreteRiccatiSolve:
LQOutputRegulatorGains gives the same result:
Compute optimal estimator gains using DiscreteRiccatiSolve:
Use LQEstimatorGains:
The optimal trajectory of the discrete approximation of a system results in a higher cost:
The optimal LQ regulation costs for the systems starting at s0:
Text
Wolfram Research (2010), DiscreteRiccatiSolve, Wolfram Language function, https://reference.wolfram.com/language/ref/DiscreteRiccatiSolve.html (updated 2014).
CMS
Wolfram Language. 2010. "DiscreteRiccatiSolve." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2014. https://reference.wolfram.com/language/ref/DiscreteRiccatiSolve.html.
APA
Wolfram Language. (2010). DiscreteRiccatiSolve. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/DiscreteRiccatiSolve.html