DiscreteWaveletTransform[data]
gives the discrete wavelet transform (DWT) of an array of data.
DiscreteWaveletTransform[data,wave]
gives the discrete wavelet transform using the wavelet wave.
DiscreteWaveletTransform[data,wave,r]
gives the discrete wavelet transform using r levels of refinement.




DiscreteWaveletTransform
DiscreteWaveletTransform[data]
gives the discrete wavelet transform (DWT) of an array of data.
DiscreteWaveletTransform[data,wave]
gives the discrete wavelet transform using the wavelet wave.
DiscreteWaveletTransform[data,wave,r]
gives the discrete wavelet transform using r levels of refinement.
Details and Options



- DiscreteWaveletTransform gives a DiscreteWaveletData object representing a tree of wavelet coefficient arrays.
- Properties of the DiscreteWaveletData dwd can be found using dwd["prop"], and a list of available properties can be found using dwd["Properties"].
- The data can be any of the following:
-
list arbitrary-rank numerical array image arbitrary Image object audio an Audio or sampled Sound object - The resulting wavelet coefficients are arrays of the same depth as the input data.
- The possible wavelets wave include:
-
BattleLemarieWavelet[…] Battle–Lemarié wavelets based on B-spline BiorthogonalSplineWavelet[…] B-spline-based wavelet CoifletWavelet[…] symmetric variant of Daubechies wavelets DaubechiesWavelet[…] the Daubechies wavelets HaarWavelet[…] classic Haar wavelet MeyerWavelet[…] wavelet defined in the frequency domain ReverseBiorthogonalSplineWavelet[…] B-spline-based wavelet (reverse dual and primal) ShannonWavelet[…] sinc function-based wavelet SymletWavelet[…] least asymmetric orthogonal wavelet - The default wave is HaarWavelet[].
- With higher settings for the refinement level r, larger-scale features are resolved.
- The default refinement level r is given by
![TemplateBox[{{{InterpretationBox[{log, _, DocumentationBuild`Utils`Private`Parenth[2]}, Log2, AutoDelete -> True], (, n, )}, +, {1, /, 2}}}, Floor] TemplateBox[{{{InterpretationBox[{log, _, DocumentationBuild`Utils`Private`Parenth[2]}, Log2, AutoDelete -> True], (, n, )}, +, {1, /, 2}}}, Floor]](Files/DiscreteWaveletTransform.en/1.png) , where
, where 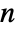 is the minimum dimension of data. »
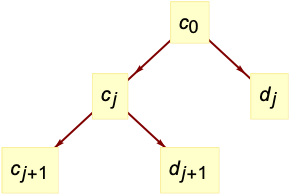
is the minimum dimension of data. » - The tree of wavelet coefficients at level
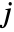 consists of coarse coefficients
consists of coarse coefficients 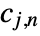 and detail coefficients
and detail coefficients 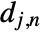 , with
, with 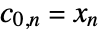 representing the input data.
representing the input data. - The forward transform is given by
 and
and  . »
. » - The inverse transform is given by
 . »
. » - The
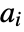 are lowpass filter coefficients and
are lowpass filter coefficients and  are highpass filter coefficients that are defined for each wavelet family.
are highpass filter coefficients that are defined for each wavelet family. - The dimensions of
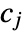 and
and 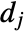 are given by
are given by ![wd_(j+1)=TemplateBox[{{{1, /, 2}, , {(, {{wd, _, j}, +, fl, -, 2}, )}}}, Ceiling] wd_(j+1)=TemplateBox[{{{1, /, 2}, , {(, {{wd, _, j}, +, fl, -, 2}, )}}}, Ceiling]](Files/DiscreteWaveletTransform.en/15.png) , where
, where 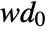 is the input data dimension and fl is the filter length for the corresponding wspec. »
is the input data dimension and fl is the filter length for the corresponding wspec. » - The following options can be given:
-
Method Automatic method to use Padding "Periodic" how to extend data beyond boundaries WorkingPrecision MachinePrecision precision to use in internal computations - The settings for Padding are the same as those available in ArrayPad.
- InverseWaveletTransform gives the inverse transform.
Examples
open all close allBasic Examples (3)
Compute a discrete wavelet transform using the HaarWavelet:
Use Normal to view all coefficients:
Use dwd[…,"Audio"] to extract coefficient signals:
Compute the inverse transform:
Transform an Image object:
Scope (36)
Basic Uses (6)
The resulting DiscreteWaveletData represents a tree of transform coefficients:
The inverse transform reconstructs the input:
Useful properties can be extracted from the DiscreteWaveletData object:
Get a full list of properties:
Get data and coefficient dimensions:
Use Normal to get all wavelet coefficients explicitly:
Also use All as an argument to get all coefficients:
Use Automatic to get only the coefficients used in the inverse transform:
Use the "TreeView" or "WaveletIndex" to find out what wavelet coefficients are available:
Extract specific coefficient arrays:
Extract several wavelet coefficients corresponding to the list of wavelet index specifications:
Extract all coefficients whose wavelet indexes match a pattern:
The Automatic coefficients are used by default in functions like WaveletListPlot:
Use a higher refinement level to increase the frequency resolution:
With a smaller refinement level, more signal energy is left in {0,0,0}:
With further refinement, {0,0,0} is resolved into further components:
Wavelet Families (10)
Compute the discrete wavelet transform using different wavelet families:
Use different families of wavelets to capture different features:
HaarWavelet (default):
Vector Data (6)
Plot the coefficients over a common horizontal axis using WaveletListPlot:
Plot against a common vertical axis:
Visualize coefficients as a function of time and refinement level using WaveletScalogram:
The coefficient indexes appear as tooltips when the mouse pointer is moved over a coefficient:
All coefficients are small except coarse coefficients {0,0,…}:
Data oscillating at the highest resolvable frequency (Nyquist frequency):
Only the first detail coefficient {1} is not small:
Data with large discontinuities:
Coarse coefficients {0,…} have the same large-scale structure as the data:
Detail coefficients are sensitive to discontinuities:
Data with both spatial and frequency structure:
Coarse coefficients {0,…} track the local mean of the data:
The first detail coefficient identifies the oscillatory region:
Matrix Data (5)
Compute a two-dimensional discrete wavelet transform:
View the tree of wavelet coefficients:
Inverse transform to get back the original signal:
Use WaveletMatrixPlot to visualize the different wavelet coefficients:
WaveletMatrixPlot of wavelet transform at a higher refinement level:
In two dimensions, the vector of filtering operations in each direction can be computed:
Interpreting these vectors as binary digit expansions results in wavelet index numbers:
Get the lowpass and highpass filters for a Haar wavelet:
The resulting 2D filters are outer products of filters in the two directions:
Wavelet transform of step data:
Data with a vertical discontinuity:
Only the vertical detail coefficients, wavelet index {…,1}, are nonzero:
Data with horizontal discontinuity:
Only the horizontal detail coefficients, wavelet index {…,2}, are nonzero:
Data with diagonal discontinuity:
Only the diagonal detail coefficients, wavelet index {…,3}, are nonzero:
Array Data (2)
Compute a three-dimensional discrete wavelet transform:
Tree view of all coefficients:
Inverse transform to get back the original signal:
Wavelet transform of a three-dimensional cross array:
Visualize wavelet coefficients:
Energy of the original data is conserved within the transformed coefficients:
Image Data (4)
Transform an Image object:
The inverse transform yields a reconstructed Image object:
Wavelet coefficients are normally given as lists of data for each image channel:
Get all coefficients as Image objects instead:
Get raw Image objects with no rescaling of color levels:
Get the inverse transform of the {0,1} coefficient as an Image object:
Plot coefficients used in the inverse transform in a hierarchical grid using WaveletImagePlot:
Image wavelet coefficients lie outside the valid range of ImageType:
"ImageFunction"->Identity gives an unnormalized image wavelet coefficient:
The color channels lie outside its valid 0 to 1 range:
By default, "ImageFunction"->ImageAdjust is used to normalize coefficients:
Sound Data (3)
Transform a Sound object:
The inverse transform yields a reconstructed Sound object:
By default, coefficients are given as lists of data for each sound channel:
Get the {0,1} coefficient as a Sound object:
Inverse transform of {0,0,1} coefficient as a Sound object:
Browse all coefficients using a MenuView:
Generalizations & Extensions (3)
DiscreteWaveletTransform works on arrays of symbolic quantities:
Inverse transform recovers the input exactly:
Specify any internal working precision:
Options (5)
Padding (2)
WorkingPrecision (3)
By default, WorkingPrecision->MachinePrecision is used:
Use higher-precision computation:
With numbers close to zero, accuracy is the better indicator of the number of correct digits:
Use WorkingPrecision->∞ for exact computation:
Applications (11)
Wavelet Compression (1)
Compress data by finding a representation with few nonzero coefficients:
SymletWavelet[n] has n vanishing moments and represents polynomials of degree n:
Count counts the number of wavelet coefficients close to 0:
Detect Discontinuities and Edges (2)
Energy Comparison (1)
Denoising (3)
Perform energy-dependent thresholding:
Computing the fraction of energy contained at each refinement level:
Set wavelet coefficients containing less than 1% energy to zero:
Perform an amplitude-dependent thresholding:
Use WaveletThreshold to perform "Universal" thresholding:
Use Stein's unbiased risk estimator smoothing:
Denoise an Image:
Perform "Soft" thresholding with threshold value "SURE" computed adaptively at each level:
Frequency Filtering (1)
Wavelet transforms can be used to filter frequencies:
To filter out the two signals, first perform a wavelet transform:
Use WaveletListPlot to visualize frequency distribution:
To filter low frequencies, keep only the coarse coefficients:
To filter high frequencies, keep only the detail coefficients:
Finance (3)
Extract the stock price trend for IBM since January 1, 2000:
The trend of the series is captured in the lowpass filter coefficients:
Thresholding all detail coefficients and inverting the series gives the trend:
Detail coefficients captured the detrended series:
Remove the trend by removing the coarse coefficients and inverting:
Study variance of returns in a financial time series:
Perform a wavelet transform using HaarWavelet and SymletWavelet:
Since the GE return series does not exhibit low-frequency oscillations, higher-scale detail coefficients do not indicate large variations from zero:
Although both filters will capture the variance of the series, they distribute it differently because of their approximate bandpass properties:
SymletWavelet isolates features in a certain frequency interval better than HaarWavelet:
Properties & Relations (15)
DiscreteWaveletPacketTransform computes the full tree of wavelet coefficients:
DiscreteWaveletTransform computes a subset of the full tree of coefficients:
DiscreteWaveletTransform coefficients halve in length with each level of refinement:
Rotated data gives different coefficients:
StationaryWaveletTransform coefficients have the same length as the original data:
Rotated data gives rotated coefficients:
Multidimensional discrete wavelet transform is related to one-dimensional packet transform:
For Haar wavelet (default) and data length ![]() , the computed coefficients are identical:
, the computed coefficients are identical:
The default refinement is given by ![]() :
:
The energy norm is conserved for orthogonal wavelet families:
The energy norm is approximately conserved for biorthogonal wavelet families:
The mean of the data is captured at the maximum refinement level of the transform:
Extract the coefficient for the maximum refinement level:
Compensate for the ![]() normalization at each refinement level:
normalization at each refinement level:
The sum of inverse transforms from individual coefficient arrays gives the original data:
Individually inverse transform each wavelet coefficient array:
The sum gives the original data:
Compute discrete wavelet coefficients for periodic data:
Define filter coefficients to have compact support:
Coarse coefficients at level ![]() are given by
are given by ![]() , with
, with ![]() :
:
Detail coefficients at level ![]() are given by
are given by ![]() :
:
Compute a partial discrete inverse wavelet transform:
Define filter coefficients to have compact support:
Coarse coefficients at level ![]() are given:
are given:
Detail coefficients at level ![]() are given:
are given:
Inverse wavelet transform at level ![]() is given by
is given by ![]() :
:
Reconstruct coarse coefficients {0,0} at refinement level ![]() :
:
Reconstruct coarse coefficients {0} at refinement level ![]() :
:
Compute the dimensions of wavelet coefficients:
At refinement level ![]() , the dimensions of wavelet coefficients are given by
, the dimensions of wavelet coefficients are given by ![]() , where
, where ![]() represents dimensions of input data:
represents dimensions of input data:
Compare ![]() dimensions with coefficient dimensions in dwd:
dimensions with coefficient dimensions in dwd:
Compute a Haar discrete wavelet transform in one dimension:
Compute {0} and {1} wavelet coefficients:
Compare with DiscreteWaveletTransform:
In two dimensions, a separate filter is applied in each dimension:
Lowpass and highpass filters for Haar wavelet:
Haar wavelet transform of matrix data:
Compare with DiscreteWaveletTransform using HaarWavelet:
Image channels are transformed individually:
Combine {0} coefficients of separately transformed image channels:
Compare with {0} coefficient of DiscreteWaveletTransform of the original image:
DWT is similar to LiftingWaveletTransform with extra coefficients needed for padding:
Possible Issues (1)
Text
Wolfram Research (2010), DiscreteWaveletTransform, Wolfram Language function, https://reference.wolfram.com/language/ref/DiscreteWaveletTransform.html (updated 2017).
CMS
Wolfram Language. 2010. "DiscreteWaveletTransform." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2017. https://reference.wolfram.com/language/ref/DiscreteWaveletTransform.html.
APA
Wolfram Language. (2010). DiscreteWaveletTransform. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/DiscreteWaveletTransform.html
BibTeX
@misc{reference.wolfram_2025_discretewavelettransform, author="Wolfram Research", title="{DiscreteWaveletTransform}", year="2017", howpublished="\url{https://reference.wolfram.com/language/ref/DiscreteWaveletTransform.html}", note=[Accessed: 17-October-2025]}
BibLaTeX
@online{reference.wolfram_2025_discretewavelettransform, organization={Wolfram Research}, title={DiscreteWaveletTransform}, year={2017}, url={https://reference.wolfram.com/language/ref/DiscreteWaveletTransform.html}, note=[Accessed: 17-October-2025]}