WOLFRAM

Wolfram Language & System Documentation Center
Wolfram Language Home Page »

ChanVeseBinarize

✖

finds a two-level segmentation of image by computing optimal contours around regions of consistent intensity in image.

✖
ChanVeseBinarize[image,marker]

uses marker to create an initial contour.

✖
ChanVeseBinarize[image,marker,{μ,ν,λ1,λ2}]

specify the Chan–Vese weights μ, ν, λ1, and λ2.

Details and Options

  • ChanVeseBinarize implements an iterative active contour method to achieve a two-level segmentation of image.
  • ChanVeseBinarize works with arbitrary 2D as well as 3D images.
  • The target region marker can be any of the following:
  • markerimagea marker image
    {pos1,pos2,…}a list of positions
    fgcolorforeground color
    {{fgcolor,bgcolor}}foreground and background colors
  • Positions posi are assumed to be in the standard image coordinate system.
  • ChanVeseBinarize uses the Euclidean distance between channel vectors to determine the similarity between pixels inside and outside of the contour.
  • The Chan–Vese segmentation of an image domain 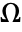into the two segments 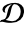and 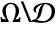with contour 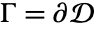minimizes the following functional 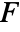of image :
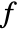  • F(c_1,c_2,Gamma)=mu Length[Gamma]+nu Area(D)+lambda_1int_DTemplateBox[{{f, -, {c, _, 1}}}, Abs]^2dxdy+lambda_2int_(Omega\D)TemplateBox[{{f, -, {c, _, 2}}}, Abs]^2dxdy
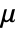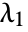  • The functional is parametrized by the length penalty , the area penalty , and level penalties and .
  • The Chan–Vese algorithm partitions image such that the first segment 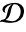will differ as little as possible from constant 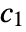and the second segment 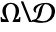will deviate as little as possible from constant 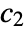. If constants and are not specified, one assumes c1=Mean[f] in 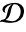, and c2=Mean[f] in .
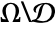  • The contour 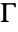between the two resulting segments 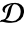and 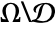will exhibit a short length for 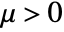, and for 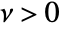the area of 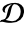will tend to be small or for tend to be large.
  • ChanVeseBinarize iteratively minimizes a functional that is a weighted sum of the contour length, the enclosed area, and the deviation between the image and the two-level segmentation.
  • The maximum number of iteration steps is given by the MaxIterations option with default setting 100.

Examples

open allclose all

Basic Examples  (2)Summary of the most common use cases

Binary segmentation of a color image:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-nzctea
Out[1]=1

Segmentation of a 3D volume:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-5lujkj
Out[1]=1

Scope  (8)Survey of the scope of standard use cases

Specify the foreground color to be used for an initial marker:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-plu7e6
Out[1]=1

Specify both foreground and background colors for creating an initial marker:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-hnccem
Out[1]=1

Use foreground edges as the marker image:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-f68zoh
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-dnqsn1
Out[2]=2

Control the area of the segmented region:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-b2beom
Out[1]=1

Increase the smoothness of the segmented region:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-d456px
Out[1]=1

Increase the length penalty when segmenting noisy images:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-eua76z
Out[1]=1

Increase the penalty for the segment to select background pixels:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-iwapol
Out[1]=1

Increase the penalty for the segment to improve the segmentation of a satellite image:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-eq472
Out[1]=1

Options  (1)Common values & functionality for each option

MaxIterations  (1)

By default, Chan–Vese segmentation iterates until convergence or until the maximum of 100 iterations:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-sng9jp
Out[2]=2

Run only a single iteration:

In[3]:=3
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-pwmve9
Out[3]=3

Applications  (3)Sample problems that can be solved with this function

Chroma key compositing:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-c9qid7
Out[2]=2

Compose the separated foreground with a different background:

In[3]:=3
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-1b9zp
Out[3]=3

Find the precise contour of a coastline in a satellite image:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-474zet
Out[1]=1

Improve text recognition of a noisy image:

In[1]:=1
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-jnj253
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0cf1s4k3uf8x3pi-vhoj6c
Out[2]=2
Wolfram Research (2010), ChanVeseBinarize, Wolfram Language function, https://reference.wolfram.com/language/ref/ChanVeseBinarize.html (updated 2014).
✖
Wolfram Research (2010), ChanVeseBinarize, Wolfram Language function, https://reference.wolfram.com/language/ref/ChanVeseBinarize.html (updated 2014).

Text

Wolfram Research (2010), ChanVeseBinarize, Wolfram Language function, https://reference.wolfram.com/language/ref/ChanVeseBinarize.html (updated 2014).

✖
Wolfram Research (2010), ChanVeseBinarize, Wolfram Language function, https://reference.wolfram.com/language/ref/ChanVeseBinarize.html (updated 2014).

CMS

Wolfram Language. 2010. "ChanVeseBinarize." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2014. https://reference.wolfram.com/language/ref/ChanVeseBinarize.html.

✖
Wolfram Language. 2010. "ChanVeseBinarize." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2014. https://reference.wolfram.com/language/ref/ChanVeseBinarize.html.

APA

Wolfram Language. (2010). ChanVeseBinarize. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/ChanVeseBinarize.html

✖
Wolfram Language. (2010). ChanVeseBinarize. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/ChanVeseBinarize.html

BibTeX

@misc{reference.wolfram_2025_chanvesebinarize, author="Wolfram Research", title="{ChanVeseBinarize}", year="2014", howpublished="\url{https://reference.wolfram.com/language/ref/ChanVeseBinarize.html}", note=[Accessed: 16-April-2025 ]}

✖
@misc{reference.wolfram_2025_chanvesebinarize, author="Wolfram Research", title="{ChanVeseBinarize}", year="2014", howpublished="\url{https://reference.wolfram.com/language/ref/ChanVeseBinarize.html}", note=[Accessed: 16-April-2025 ]}

BibLaTeX

@online{reference.wolfram_2025_chanvesebinarize, organization={Wolfram Research}, title={ChanVeseBinarize}, year={2014}, url={https://reference.wolfram.com/language/ref/ChanVeseBinarize.html}, note=[Accessed: 16-April-2025 ]}

✖
@online{reference.wolfram_2025_chanvesebinarize, organization={Wolfram Research}, title={ChanVeseBinarize}, year={2014}, url={https://reference.wolfram.com/language/ref/ChanVeseBinarize.html}, note=[Accessed: 16-April-2025 ]}