WolframのWebサイトのコンテンツとインタラクトしたりフォームを送信したりするためには，JavaScriptを有効にしてください．有効にする方法

InverseWishartMatrixDistribution

InverseWishartMatrixDistribution[ν,Σ]

自由度 ν，共分散行列 Σ の逆Wishart 行列分布を表す．

詳細

逆Wishart行列分布における対称行列の確率密度は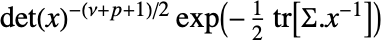に比例する．ただし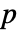，は行列 Σ の大きさである．
InverseWishartMatrixDistribution[ν,Σ]として分布している行列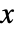について，逆行列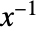はWishartMatrixDistribution[ν,Σ^-1]として分布する．
共分散行列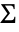は次元が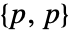の正定値対称行列でよく，ν は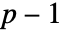より大きい任意の実数でよい．
InverseWishartMatrixDistributionは，MatrixPropertyDistribution，EstimatedDistribution，RandomVariate等の関数とともに使うことができる．

例題

すべて開くすべて閉じる

例 (3)

擬似ランダム行列を生成する：

これが正定値行列かどうかチェックする：

MatrixPropertyDistributionを使って逆Wishartランダム行列の固有値をサンプルする：

平均と分散：

スコープ (6)

単一の擬似ランダム行列を生成する：

擬似ランダム行列の集合を生成する：

拡張精度でサンプルする：

統計特性を数値計算する：

最大行列固有値の期待値を数値近似する：

分布母数推定：

サンプルデータから分布母数を推定する：

両分布のLogLikelihoodを比較する：

歪度：

特性と関係 (3)

（およびは独立ガウス(Gauss)ベクトルおよびWishart行列）はHotellingTSquareDistributionに従う：

MatrixPropertyDistributionを使って式をサンプルする：

逆Wishartランダム行列の任意の直交要素はスケールされた逆カイ二乗分布に従う：

直交要素は独立ではない：

任意の非零ベクトルおよびスケール行列を持つ逆Wishart行列について，はカイ二乗分布に従う：

トップへ