Enable JavaScript to interact with content and submit forms on Wolfram websites. Learn how

Wolfram Language & System Documentation Center

SphericalHarmonicY

SphericalHarmonicY

SphericalHarmonicY[l,m,θ,ϕ]

gives the spherical harmonic .

Details

Mathematical function, suitable for both symbolic and numerical manipulation.
The spherical harmonics are orthonormal with respect to integration over the surface of the unit sphere.
For 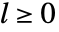, 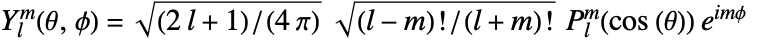where 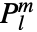is the associated Legendre function.
For , .
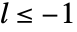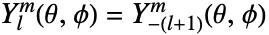For certain special arguments, SphericalHarmonicY automatically evaluates to exact values.
SphericalHarmonicY can be evaluated to arbitrary numerical precision.
SphericalHarmonicY automatically threads over lists.

Examples

open all close all

Basic Examples (5)

Evaluate symbolically:

Plot over a subset of the reals:

Plot over a subset of the complexes:

Series expansion at the origin:

Series expansion at Infinity:

Scope (36)

Numerical Evaluation (6)

Evaluate numerically:

Evaluate to high precision:

The precision of the output tracks the precision of the input:

Complex number inputs:

Evaluate efficiently at high precision:

Compute average-case statistical intervals using Around:

Compute the elementwise values of an array:

Or compute the matrix SphericalHarmonicY function using MatrixFunction:

Specific Values (4)

Evaluate SphericalHarmonicY symbolically for integer orders:

Evaluate SphericalHarmonicY symbolically for noninteger orders:

Evaluate SphericalHarmonicY symbolically for :

SphericalHarmonicY for symbolic l and m:

Find the first positive maximum of SphericalHarmonicY[2,2,θ,Pi/2]:

Visualization (3)

Plot the SphericalHarmonicY function for various orders:

Plot the real part of :

Plot the imaginary part of :

Plot the absolute value of the SphericalHarmonicY function in three dimensions:

Function Properties (13)

For integer and , is defined for all complex and :

For , it is defined as a real function for all real and :

For other values of , it is typically not defined as a real function:

The real range of :

The range for complex values:

is an even function with respect to for even-order :

It is an odd function with respect to for odd-order :

SphericalHarmonicY is a periodic function with respect to θ and ϕ:

SphericalHarmonicY threads elementwise over lists:

is an analytic function of and for integer and :

For , it is analytic over the reals:

is neither non-decreasing nor non-increasing as a function of :

is not injective:

is not surjective:

is neither non-positive nor non-negative:

does not have either a singularity or a discontinuity over the complexes for integer and :

For , it is nonsingular over the reals as well:

is neither convex nor concave:

TraditionalForm formatting:

Differentiation (3)

First derivative with respect to ϕ:

First derivative with respect to θ:

Higher derivatives with respect to θ:

Plot the absolute values of the higher derivatives of with respect to :

Formula for the derivative with respect to :

Integration (3)

Compute the indefinite integral using Integrate:

Verify the anti-derivative:

Definite integral:

More integrals:

Series Expansions (4)

Find the Taylor expansion using Series:

General term in the series expansion using SeriesCoefficient:

Taylor expansion at a generic point:

Generalizations & Extensions (1)

SphericalHarmonicY can be applied to a power series:

Applications (2)

The spherical harmonics are eigenfunctions of the Laplacian on the sphere:

The eigenvalues equal :

Plot hydrogen orbital densities for quantum numbers , , :

Plot :

Properties & Relations (2)

Use FunctionExpand to expand SphericalHarmonicY[n,m,θ,ϕ] for half-integers and :

Re-express spherical harmonics in Cartesian coordinates:

Top