gives a TransformationFunction that represents an affine transform that maps r to m.r.
AffineTransform[{m,v}]
gives an affine transform that maps r to m.r+v.


AffineTransform
gives a TransformationFunction that represents an affine transform that maps r to m.r.
AffineTransform[{m,v}]
gives an affine transform that maps r to m.r+v.
Details
- AffineTransform gives a TransformationFunction that can be applied to vectors.
- For ordinary affine transforms in
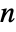 dimensions, m is an
dimensions, m is an 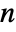 ×
×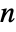 matrix.
matrix. - AffineTransform in general supports
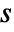 ×
× matrices for transformations in
matrices for transformations in  dimensions.
dimensions.
Examples
open all close allBasic Examples (2)
Scope (3)
Applications (5)
Iterated Function Systems (3)
Image Transformations (2)
Properties & Relations (3)
Related Guides
History
Text
Wolfram Research (2007), AffineTransform, Wolfram Language function, https://reference.wolfram.com/language/ref/AffineTransform.html.
CMS
Wolfram Language. 2007. "AffineTransform." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/AffineTransform.html.
APA
Wolfram Language. (2007). AffineTransform. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/AffineTransform.html
BibTeX
@misc{reference.wolfram_2025_affinetransform, author="Wolfram Research", title="{AffineTransform}", year="2007", howpublished="\url{https://reference.wolfram.com/language/ref/AffineTransform.html}", note=[Accessed: 28-October-2025]}
BibLaTeX
@online{reference.wolfram_2025_affinetransform, organization={Wolfram Research}, title={AffineTransform}, year={2007}, url={https://reference.wolfram.com/language/ref/AffineTransform.html}, note=[Accessed: 28-October-2025]}