 |
BUILT-IN MATHEMATICA SYMBOL
Interpolation
Interpolation[{f1, f2, ...}]
constructs an interpolation of the function values 
, assumed to correspond to x values 1, 2, ... .
Interpolation[{{x1, f1}, {x2, f2}, ...}]
constructs an interpolation of the function values 
corresponding to x values 
.
Interpolation[{{{x1, y1, ...}, f1}, {{x2, y2, ...}, f2}, ...}]
constructs an interpolation of multidimensional data.
Interpolation[{{{x1, ...}, f1, df1, ...}, ...}]
constructs an interpolation that reproduces derivatives as well as function values.
Interpolation[data, x]
find an interpolation of data at the point x.
Details and Options
- Interpolation returns an InterpolatingFunction object, which can be used like any other pure function.
- The interpolating function returned by Interpolation[data] is set up so as to agree with data at every point explicitly specified in data.
- The function values
can be real or complex numbers, or arbitrary symbolic expressions. - The
can be lists or arrays of any dimension. - The function arguments
, 
, etc. must be real numbers. - Different elements in the data can have different numbers of derivatives specified.
- For multidimensional data, the n
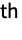derivative can be given as a tensor with a structure corresponding to D[f, {{x, y, ...}, n}]. - Partial derivatives not specified explicitly can be given as Automatic.
- Interpolation works by fitting polynomial curves between successive data points.
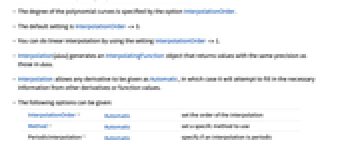
- The degree of the polynomial curves is specified by the option InterpolationOrder.
- The default setting is InterpolationOrder->3.
- You can do linear interpolation by using the setting InterpolationOrder->1.
- Interpolation[data] generates an InterpolatingFunction object which returns values with the same precision as those in data.
- Interpolation allows any derivative to be given as Automatic, in which case it will attempt to fill in the necessary information from other derivatives or function values.
- Interpolation supports a Method option. Possible settings include
for spline interpolation and 
for Hermite interpolation.
New in 2 | Last modified in 7
