BUILT-IN WOLFRAM LANGUAGE SYMBOL

ContinuousWaveletTransform

ContinuousWaveletTransform[{x₁,x₂,…}]
gives the continuous wavelet transform of a list of values .

ContinuousWaveletTransform[data,wave]
gives the continuous wavelet transform using the wavelet wave.

ContinuousWaveletTransform[data,wave,{noct,nvoc}]
gives the continuous wavelet transform using noct octaves with nvoc voices per octave.

ContinuousWaveletTransform[sound,…]
gives the continuous wavelet transform of sampled sound.

Details and Options Details and Options

ContinuousWaveletTransform gives a ContinuousWaveletData object.
Properties of the ContinuousWaveletData cwd can be found using cwd["prop"]. A list of available properties can found using cwd["Properties"].
The resulting wavelet coefficients are arrays of the same dimensions as the input data.
The possible wavelets wave include:

	MorletWavelet[…]	Morlet cosine times Gaussian
	GaborWavelet[…]	complex Morlet wavelet
	DGaussianWavelet[…]	derivative of Gaussian
	MexicanHatWavelet[…]	second derivative of Gaussian
	PaulWavelet[…]	Paul wavelet

The default wave is MexicanHatWavelet[].
The default value for noct is given by $TemplateBox[{{InterpretationBox[{log, _, DocumentationBuild`Utils`Private`Parenth[2]}, Log2, AutoDelete -> True], (, {n, /, 2}, )}}, Floor]$ , where 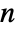is the length of the input. »
The default value for nvoc is 4.
The continuous wavelet transform of a function 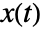is given by $w(u,s)=1/(sqrt(s))int_(-infty)^inftyx(t) TemplateBox[{psi}, Conjugate]((t-u)/s)dt$ .
The continuous wavelet transform of a uniformly sampled sequence 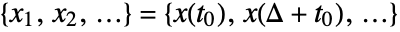is given by $w(u,s)=1/(sqrt(s))sum_(k=1)^nx_k TemplateBox[{psi}, Conjugate]((Delta (k-u))/s)$ .
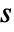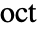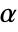The scaling parameter is given by equal-tempered scale where is the octave number, the voice number, and the smallest wavelet scale.
For each scale 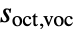, the ContinuousWaveletTransform computes the wavelet coefficients .
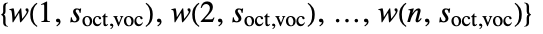The following options can be given:

Padding	None	how to extend data beyond boundaries
SampleRate	Automatic	samples per unit
WaveletScale	Automatic	smallest resolvable scale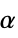
WorkingPrecision	MachinePrecision	precision to use in internal computations

Padding pads the input data to the next higher power of 2 to reduce boundary effects. The settings for Padding are the same as for the padding argument used in ArrayPad.
InverseContinuousWaveletTransform gives the inverse transform.