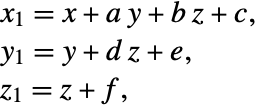Diophantine Polynomial Systems
| Introduction | Multivariate Nonlinear Systems |
| Linear Systems | Options |
| Univariate Systems | References |
| Bivariate Systems |
Introduction
A Diophantine polynomial system is an expression constructed with polynomial equations and inequalities
combined using logical connectives and quantifiers
where the variables represent integer quantities.
An occurrence of a variable 
inside 
or 
is called a bound occurrence; any other occurrence of 
is called a free occurrence. A variable 
is called a free variable of a polynomial system if the system contains a free occurrence of 
. A Diophantine polynomial system is quantifier-free if it contains no quantifiers. A decision problem is a system with all variables existentially quantified, that is, a system of the form
where 
are all variables in 
. The decision problem (1) is equivalent to True or False, depending on whether the quantifier-free system of polynomial equations and inequalities 
has integer solutions.
An example of a Diophantine polynomial system is
Goldbach's conjecture [1], formulated in 1742 and still unproven, asserts that system (2) is equivalent to True. This suggests that the Wolfram Language may not be able to solve arbitrary Diophantine polynomial systems. In fact, Matiyasevich's solution of Hilbert's tenth problem [2] shows that no algorithm can be constructed that would solve arbitrary Diophantine polynomial systems, not even quantifier-free systems or decision problems. Nevertheless, the Wolfram Language functions Reduce, Resolve, and FindInstance are able to solve several reasonably large classes of Diophantine systems. This tutorial describes these classes of systems and methods used by the Wolfram Language to solve them. The methods are presented in the order in which they are used. The tutorial also covers options affecting the methods used and how they operate.
Linear Systems
Systems of Linear Equations
Conjunctions of linear Diophantine equations are solvable for an arbitrary number of variables. The Wolfram Language uses a method based on the computation Hermite normal form of matrices, available in the Wolfram Language directly as HermiteDecomposition. The result may contain new unrestricted integer parameters. If the equations are independent, the number of parameters is equal to the difference between the number of variables and the number of equations.
Frobenius Equations
A Frobenius equation is an equation of the form
where 
are positive integers, 
is an integer, and the coordinates 
of solutions are required to be non‐negative integers.
For finding solution instances of Frobenius equations the Wolfram Language uses a fast algorithm based on the computation of the critical tree in the Frobenius graph [11]. The algorithm applies when the smallest of 
does not exceed the value of the MaxFrobeniusGraph system option (the default is 1,000,000). Otherwise the more general methods for solving bounded linear systems are used. Functions FrobeniusSolve and FrobeniusNumber provide specialized functionality for solving Frobenius equations and computing Frobenius numbers.
Bounded Systems of Linear Equations and Inequalities
If a real solution set of a system of linear equations and inequalities is a bounded polyhedron, the system has finitely many integer solutions. To find the solutions, the Wolfram Language uses the following procedure.
You may assume the system has the form 
, where 
is a 
integer matrix, 
is a length 
integer vector, 
is an 
integer matrix, and 
is a length 
integer vector. First, the method for solving systems of linear equations is used to find an integer vector 
such that 
and a 
integer matrix N whose rows generate the null space of 
. The integer solution set of 
is equal to 
. Put 
and 
. The integer solution set of 
is equal to 
, where 
is the integer solution set of 
. To improve efficiency of finding the set 
, the Wolfram Language simplifies 
using LatticeReduce, obtaining 
. Note that if the columns of 
are linearly dependent, 
has no solutions (otherwise it would have infinitely many solutions, which contradicts the assumptions). Hence you may assume that 
has linearly independent columns and so 
has 
columns. Put 
. Lattice reduction techniques are also used to find a small vector 
in the lattice 
. Let 
be such that 
. The set 
can be computed from the set 
of all 
such that 
using the formula 
.
To find the set 
a simple recursive algorithm can be used. The algorithm finds the bounds on the first variable using LinearProgramming and, for each integer value 
between the bounds, calls itself recursively with the first variable set to 
. This algorithm is used when the system option BranchLinearDiophantine is set to False. With the default setting True a hybrid algorithm combining the recursive algorithm and a branch-and-bound-type algorithm is used. At each level of the recursion, the recursion is continued for the "middle" values of the first variable (defined as a projection of the set of points contained in the real solution set together with a unit cube) while the remaining parts of the real solution set are searched for integer solutions using the branch-and-bound type algorithm. FindInstance finds the single element of 
it needs using a branch-and-bound type algorithm.
There are two system options, BranchLinearDiophantine and LatticeReduceDiophantine, that allow you to control the exact algorithm used. In some cases changing the values of these options may greatly improve the performance of Reduce.
The Wolfram Language enumerates the solutions explicitly only if the number of integer solutions of the system does not exceed the maximum of the 
th power of the value of the system option DiscreteSolutionBound, where 
is the dimension of the solution lattice of the equations, and the second element of the value of the system option ExhaustiveSearchMaxPoints.
, and Reduce can enumerate the solutions explicitly:Arbitrary Systems of Linear Equations and Inequalities
Quantifier-free systems of linear Diophantine equations and inequalities are solvable for an arbitrary number of variables. The system is written in the disjunctive normal form, that is, as a disjunction of conjunctions, and each conjunction is solved separately. If a conjunction contains only equations, the method for solving systems of linear equations is used. If the difference between the number of variables and the number of equations is at most one, the Wolfram Language solves the equations using the method for solving systems of linear equations, and if the solution contains at most one free parameter (which is true in the generic case), back substitutes the solution into the inequalities to determine inequality restrictions for the parameter. For all other quantifier-free systems of linear Diophantine equations and inequalities the Wolfram Language uses the algorithm described in [3], with some linear-programming-based improvements for handling bounded variables. The result may contain new non‐negative integer parameters, and the number of new parameters may be larger than the number of variables.
Univariate Systems
Univariate Equations
To find integer solutions of univariate equations the Wolfram Language uses a variant of the algorithm given in [4] with improvements described in [5]. The algorithm can find integer solutions of polynomials of much higher degrees than can be handled by real root isolation algorithms and with much larger free terms than can be handled by integer factorization algorithms.
Systems of Univariate Equations and Inequalities
Systems of univariate Diophantine equations and inequalities are written in the disjunctive normal form, and each conjunction is solved separately. If a conjunction contains an equation, the method for solving univariate equations is used, and the solutions satisfying the remaining equations and inequalities are selected.
Conjunctions containing only inequalities are solved over the reals. Integer solutions in the resulting real intervals are given explicitly if their number in the given interval does not exceed the value of the system option DiscreteSolutionBound. The default value of the option is 10. For intervals containing more integer solutions, the solutions are represented implicitly.
Bivariate Systems
Quadratic Equations
The Wolfram Language can solve arbitrary quadratic Diophantine equations in two variables. The general form of such an equation is
If 
, where
and 
are linear polynomials, the equation (1) is equivalent to 
, and methods for solving linear Diophantine equations are used. For irreducible polynomials 
, the algorithms used and the form of the result depend on the determinant 
of the quadratic form. The algorithms may use integer factorization and hence the correctness of the results depends on the correctness of the probabilistic primality test used by PrimeQ.
If 
and 
is an integer, then 
, where
and 
are linear polynomials and 
. In this case, the equation (1) is equivalent to the disjunction of linear systems 
, for all divisors 
of 
. Each of the linear systems has one solution over the rationals, hence the equation (1) has a finite number of integer solutions.
If 
and 
is not an integer, then the equation (1) is a Pell-type equation. Methods for solving such equations have been developed since the 18 th century and can be constructed based on [6] and [7] (though these books do not contain a complete description of the algorithm). The solution set is empty or infinite, parametrized by an integer parameter appearing in the exponent.
, where 
is not a square. Solutions to Pell equations with small coefficients can be quite complicated:
If 
, set 
, 
, and 
. Since 
, 
and 
are nonzero integers, and 
. Then
Set 
and 
. Then the equation (1) is equivalent to
Suppose 
. If the equation (1) had integer solutions, 
would have integer solutions in 
, and so 
would be a product of two linear polynomials. Since here 
is irreducible, the equation (1) has no integer solutions.
If 
, then the equation (2) implies
If the modular equation (3) has no solutions in 
, the equation (1) has no integer solutions. (If 
, the modular equation (3) has one solution, 
.) Otherwise 
, for some solution 
of the modular equation (3). Replacing 
in the equation (2) and solving the resulting linear equation for 
gives
Note that since 
satisfies the modular equation (3), the division in the last term of (4) gives an integer result. Since 
and 
, 
. Taking the equation (4) and the fact that 
into account gives
Therefore, the full solution of the equation (1) of parabolic type consists of one-parameter solution families given by equations (4) and (5) for each solution 
of the modular equation (3), for which 
is an integer.
If 
, the solutions of equation (1) are integer points on an ellipse. Since an ellipse is a bounded set, the number of solutions must be finite. An obvious algorithm for finding integer points would be to compute the solutions for 
for each of the finite number of possible integer values of 
. This, however, would be prohibitively slow for larger ellipses. The Wolfram Language uses a faster algorithm described in [8].
possible positive integer values of 
, so the obvious algorithm would not be practical for this ellipse:Thue Equations
A Thue equation is a Diophantine equation of the form
where 
is an irreducible homogeneous form of degree 
The number of solutions of Thue equations is always finite. The Wolfram Language can in principle solve arbitrary Thue equations, though the time necessary to find the solutions lengthens very fast with degree and coefficient size. The hardest part of the algorithm is computing a bound on the size of solutions. The Wolfram Language uses an algorithm based on the Baker–Wustholz theorem to find the bound [9]. If the input contains inequalities that provide a reasonable size bound on solutions, the Wolfram Language can compute the solutions much faster.
coordinate. Without the bound on the solution size, Reduce did not finish in 1000 seconds:Multivariate Nonlinear Systems
Systems Solvable with the Modular Sieve Method
The Wolfram Language uses a variant of the modular sieve method (see e.g. [9]). The method may prove that a system has no solutions in integers modulo an integer 
, and therefore, it has no integer solutions. Otherwise, it may find a solution with small integer coordinates or prove that the system has no integer solutions with all coordinates between 
and 
. The number of candidate solution points that the sieve method is allowed to test is controlled by the system option SieveMaxPoints.
Systems with More than One Equation
If a Diophantine polynomial system contains more than one equation, the Wolfram Language uses GroebnerBasis in an attempt to reduce the problem to a sequence of simpler problems.
The Wolfram Language attempts to solve an element of the Gröbner basis that depends on the minimal number of the initial variables. If the number of solutions is finite, then for each solution the Wolfram Language substitutes the computed values and attempts to solve the obtained system for the remaining variables.
and 
. For each of the solutions, the second equation becomes a univariate equation in 
. The four univariate equations have a total of two integer solutions:
and 
with the values computed from the first equation, the second equation becomes a Pell equation:This heuristic applies to systems with Gröbner bases of the form
In this case, the Wolfram Language solves the system of congruences
The solutions are represented using new integer parameters. These are substituted into the equation 
and the inequalities present in the original system, and the Wolfram Language attempts to solve the so-obtained systems for the new parameters.
Sums of Squares
The Wolfram Language can solve equations of the form
using the algorithm described in [10]. For multivariate quadratic equations, the Wolfram Language attempts to find an affine transformation that puts the equation in the form (2). A heuristic method based on CholeskyDecomposition is used for this purpose. However, the method may fail for some equations that can be represented in the form (2).
To find a single solution of (2) FindInstance uses an algorithm based on [12].
Pythagorean Equation
The Wolfram Language knows the solution to the Pythagorean equation
For quadratic equations in three variables, the Wolfram Language attempts to find a transformation of the form
transforming the equation to the Pythagorean equation.
Equations with Reducible Nonconstant Parts
If the sum of nonconstant terms in an equation factors, the Wolfram Language uses the formula
to reduce the equation to a disjunction of pairs of equations with lower degrees. Note that this reduction depends on the ability to find all divisors of 
, hence the correctness of the results depends on the correctness of the probabilistic primality test used by PrimeQ.
Equations with a Linear Variable
The Wolfram Language attempts to solve Diophantine systems of the form
where 
is a conjunction of inequalities or True, by reducing them to
The first part of the system (3) is solved using the method for solving systems with more than one equation. The Wolfram Language recognizes three cases when the second part of the system (3) is solvable. If 
, the solution is given by 
and by the restrictions on 
obtained by solving the inequalities 
. Nonlinear systems of inequalities are solved using CylindricalDecomposition. If 
for an integer constant 
, the solution of the second part of the system (3) is given by 
and by the restrictions on 
obtained by solving the congruence 
and then solving the inequalities 
for each solution of the congruence. If 
is nonconstant, the Wolfram Language can solve the second part of the system (3) if 
. Since the Wolfram Language factors all equations at the preprocessing stage, 
and 
can be assumed to be relatively prime. Then
for an integer 
and polynomials 
and 
with integer coefficients and 
. If 
is an integer, then 
is an integer, and so 
or 
. Since 
, the last condition is satisfied only by a finite number of integers 
. Hence the solutions of the second part of the system (3) can be selected from a finite number of solution candidates.
Additionally, the Wolfram Language uses the following heuristic to detect cases when the system (3) has no solutions. If there is an integer 
, such that 
is always divisible by 
, and 
is never divisible by 
, then the system (3) has no solutions. Candidates for 
are found by computing the GCD of the values of 
at several points.
The last two methods use exhaustive search over finite sets of points. The allowed number of search points is controlled by the system option SieveMaxPoints.
is always divisible by 5, and 
is never divisible by 5:Systems with Empty or Bounded Real Solution Sets
If a Diophantine polynomial system is not solved by any other methods, the Wolfram Language solves the system over the reals using the Cylindrical Algebraic Decomposition (CAD) algorithm. If the system has no real solutions, then clearly it has no integer solutions. If the real solution set is bounded, then the number of integer solutions is finite. In principle, all the integer solutions can be found in this case from a cylindrical decomposition. Namely, for each cylinder, you enumerate all possible integer values of the first coordinate, then for each value of the first coordinate, you enumerate all possible integer values of the second coordinate, and so on. However, for large bounded solution sets this method could lead to a huge number of points to try. Therefore, the Wolfram Language has a bound on the number of explicitly enumerated integer solutions in a real interval. For systems for which the real solution set is unbounded or bounded but large, the solution is represented implicitly by returning the CAD and a condition that all variables are integers. Note that for multivariate systems such an implicit representation may not even be enough to tell whether integer solutions exist. This should be expected, given Matiyasevich's solution of Hilbert's tenth problem [2].
. After adding inequalities to eliminate this cube, Reduce then recognizes that this equation has no solutions anywhere:Equations of the Form 
The Wolfram Language attempts to solve Diophantine systems of the form
where 
is a conjunction of inequalities or True, by transforming them to
The resulting system (4) may, or may not, be easier to solve. Systems exist for which this transformation could be applied recursively arbitrarily many times; therefore, the Wolfram Language uses a recursion bound to ensure the heuristic terminates.
Systems Solvable by Exhaustive Search
For systems containing explicit lower and upper bounds on all variables, the Wolfram Language uses exhaustive search to find solutions. The bounds of the search are specified by the value of the system option ExhaustiveSearchMaxPoints. The option value should be a pair of integers (the default is 
). If the number of integer points within the bounds does not exceed the first integer, the exhaustive search is used instead of any solution methods other than univariate polynomial solving. Otherwise, if the number of integer points within the bounds does not exceed the second integer, the exhaustive search is performed after all other methods fail.
Options
The Wolfram Language functions for solving Diophantine polynomial systems have a number of options that control the way they operate. This tutorial gives a summary of these options.
option name | default value | |
| GeneratedParameters | C | specifies how the new parameters generated to represent solutions should be named |
Reduce options affecting the behavior for Diophantine polynomial systems.
GeneratedParameters
To represent infinite solutions of some Diophantine systems, Reduce needs to introduce new integer parameters. The names of the new parameters are specified by the option GeneratedParameters. With GeneratedParameters->f, the new parameters are named f[1], f[2], … .
ReduceOptions Group of System Options
Here are the system options from the ReduceOptions group that may affect the behavior of Reduce, Resolve, and FindInstance for Diophantine polynomial systems. The options can be set with
SetSystemOptions["ReduceOptions"->{"option name"->value}].
option name | default value | |
| "BranchLinearDiophantine" | True | whether Reduce should use a branch-and-bound-type algorithm to compute solutions of bounded systems of linear Diophantine inequalities |
| "DiscreteSolutionBound" | 10 | the bound on the number of explicitly enumerated integer solutions in a real interval |
| "ExhaustiveSearchMaxPoints" | {1000,10000} | the maximal number of integer points within variable bounds for which the exhaustive search is used before and after all other solution methods |
| "ImplicitIntegerSolutions" | Automatic | whether Reduce should be allowed to return real solutions with Element conditions when it cannot find explicit integer solutions |
| "LatticeReduceDiophantine" | True | whether LatticeReduce should be used to preprocess bounded systems of linear Diophantine inequalities |
| "MaxFrobeniusGraph" | 1000000 | the maximal size of the smallest coefficient in a Frobenius equation for which FindInstance computes the critical tree in the Frobenius graph |
| "SieveMaxPoints" | {10000,1000000} | the maximal number of points at which the modular sieve method evaluates the system before and after all other solution methods |
ReduceOptions group options affecting the behavior of Reduce, Resolve, and FindInstance for Diophantine polynomial systems.
The value of the system option BranchLinearDiophantine specifies which variant of the algorithm should be used in the final stage of solving bounded linear systems. With the default setting "BranchLinearDiophantine"->False, a simple recursive enumeration is used. With "BranchLinearDiophantine"->True, Reduce uses a hybrid method combining a branch-and-bound-type algorithm and a simple recursive enumeration.
and three randomly generated inequalities 
in seven variables inside a simplex bounded by 
. Here the speed difference between the algorithms is smaller:The value of the system option DiscreteSolutionBound specifies whether integer solutions in a real interval 
should be enumerated explicitly or represented implicitly as 
. With DiscreteSolutionBound->n, the integer solutions in the given real interval are enumerated explicitly if their number does not exceed n. The default value of the option is 10.
The value of DiscreteSolutionBound also affects the solving of bounded linear systems and other Diophantine systems with finitely many solutions. When you need an explicit enumeration of a large number of solutions in a bounded region, set DiscreteSolutionBound to Infinity.
The system option ExhaustiveSearchMaxPoints specifies the maximal number of search points used by the exhaustive search method. The option value should be a pair of integers (the default is 
). If the number of integer points within the bounds does not exceed the first integer, the exhaustive search is used instead of any solution methods other than univariate polynomial solving. Otherwise, if the number of integer points within the bounds does not exceed the second integer, the exhaustive search is performed after all other methods fail.
makes Reduce use the exhaustive search first. In this example the search is much slower than the Pell equation solver:The value of the system option DiscreteSolutionBound specifies whether integer solutions in a real interval 
should be enumerated explicitly or represented implicitly as 
. With DiscreteSolutionBound->n, the integer solutions in the given real interval are enumerated explicitly if their number does not exceed n. The default value of the option is 10.
The value of the system option LatticeReduceDiophantine specifies whether LatticeReduce should be used to preprocess systems of bounded linear inequalities. The use of LatticeReduce is important for systems of inequalities describing polyhedra whose projections on some nonaxial lines are much smaller than their projections on the axes. However, there are systems for which LatticeReduce, instead of simplifying the problem, makes it significantly harder.
but whose projection on the line 
has size one:
, which bound solutions to a reasonably small size polyhedron, combined with a set of relatively complicated inequalities 
. For such systems, using LatticeReduce tends to increase the timing:The system option MaxFrobeniusGraph specifies the maximal size of the smallest coefficient in a Frobenius equation for which FindInstance uses an algorithm based on the computation of the critical tree in the Frobenius graph [11]. Otherwise, the more general methods for solving bounded linear systems are used. Unlike the general method for solving bounded linear systems, the method based on the computation of the Frobenius graph depends very little on the number of variables, hence it is the faster choice for equations with many variables. On the other hand, the method requires storing a graph of the size of the smallest coefficient, so for large coefficients it may run out of memory.
, FindInstance by default uses the general method for solving bounded linear systems. For this example the method is relatively slow but uses little memory. The kernel has been restarted to show the memory usage by the current example:
The system option SieveMaxPoints specifies the maximal number of search points used by the modular sieve method and by searches used in solving equations with a linear variable. The value of the option is a pair 
. Search involving up to 
points is tried before some of the slower solving methods. For decision problems, another search involving up to 
points is tried after all other methods fail. The default value of the option is 
.
References
in Coprime Integers 
and 
." Mathematics of Computation 55 (1990): 327–343.