WaveletImagePlot[dwd]
plots the basis tree of wavelet image coefficients in the DiscreteWaveletData dwd.
WaveletImagePlot[dwd,r]
plots coefficients up to refinement level r.
WaveletImagePlot[dwd,r,ifunc]
applies the image function ifunc to coefficients and wavelet indexes before plotting.


WaveletImagePlot
WaveletImagePlot[dwd]
plots the basis tree of wavelet image coefficients in the DiscreteWaveletData dwd.
WaveletImagePlot[dwd,r]
plots coefficients up to refinement level r.
WaveletImagePlot[dwd,r,ifunc]
applies the image function ifunc to coefficients and wavelet indexes before plotting.
Details and Options


- WaveletImagePlot assembles a single image using a pyramid of images.
- WaveletImagePlot arranges coefficients in the same way as WaveletMatrixPlot.
- WaveletImagePlot[dwd] is equivalent to WaveletImagePlot[dwd,Automatic], corresponding to the basis of coefficients used in the InverseWaveletTransform.
- The wavelet indices used in the Automatic setting are given by dwd["BasisIndex"].
- The dwd should be generated from image data and produced by DiscreteWaveletTransform, DiscreteWaveletPacketTransform, or LiftingWaveletTransform.
- WaveletImagePlot[dwd,r] plots basis coefficient images up to refinement level r when dwd comes from DiscreteWaveletTransform or LiftingWaveletTransform.
- WaveletImagePlot[dwd,r] plots all coefficient images at refinement level r when dwd comes from DiscreteWaveletPacketTransform.
- In WaveletImagePlot[dwd,r,ifunc], each ifunc[coefi,windi] should yield an Image with the same dimensions as coefi.
- WaveletImagePlot has the same options as Image, with the following additions and changes: [List of all options]
-
BaseStyle Black what base color to use between images PlotLayout Automatic what layout to use for the plot - Possible settings for PlotLayout include: "Pyramid" and "Grid".
- BaseStyle is used to specify the color of pixels to use at coefficient boundaries.
- With the setting Method->"InverseTransform", the inverse transform of each coefficient array will be plotted.
- WaveletImagePlot returns an Image object.
-
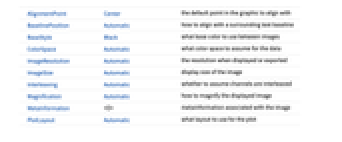
AlignmentPoint Center the default point in the graphic to align with BaselinePosition Automatic how to align with a surrounding text baseline BaseStyle Black what base color to use between images ColorSpace Automatic what color space to assume for the data ImageResolution Automatic the resolution when displayed or exported ImageSize Automatic display size of the image Interleaving Automatic whether to assume channels are interleaved Magnification Automatic how to magnify the displayed image MetaInformation <||> metainformation associated with the image PlotLayout Automatic what layout to use for the plot
List of all options
Examples
open all close allBasic Examples (2)
Scope (9)
Data (5)
DiscreteWaveletTransform of image data:
Plot image wavelet coefficients in a hierarchical grid:
The plotted coefficients are the Automatic coefficients used in the inverse transform:
Use WaveletImagePlot[dwd,r] to plot coefficients only up to refinement level r:
The hierarchical layout corresponds to the tree structure of the wavelet coefficients:
DiscreteWaveletPacketTransform of image data:
The DiscreteWaveletData object contains a full tree of coefficients at each level:
Default Automatic coefficients for packet transform correspond to the highest refinement level:
WaveletBestBasis computes a different Automatic tree of coefficients:
Specify an image function f to apply to wavelet coefficients before plotting:
By default, ImageAdjust[ImageApply[Abs,#]]& is applied:
Options (4)
ImageSize (1)
Specify the size of the displayed image:
The pixel dimensions of the image are not affected by ImageSize:
Method (2)
Inverse transform each coefficient before plotting:
Inverse transform coefficients from stationary wavelet transform:
Inverse transform coefficients from lifting wavelet transform:
Choose which channel to plot in multichannel Image:
Properties & Relations (6)
WaveletImagePlot returns an Image object:
Pixel dimensions of generated images depend on padding, wavelet, and refinement level:
The generated image is generally larger than the original data:
WaveletImagePlot plots image wavelet coefficients in a hierarchical grid layout:
dwd[…,"Image"] gives each coefficient as a separate image:
WaveletImagePlot plots the Automatic coefficients used in the inverse transform:
WaveletBestBasis selects a different default tree of coefficients:
WaveletMatrixPlot plots matrix wavelet coefficients in a hierarchical grid:
WaveletScalogram plots vector coefficients with numerical magnitude indicated by color:
WaveletListPlot plots vector coefficients with a common horizontal or vertical axis:
Possible Issues (3)
Generated images may be saturated if a custom image function f is specified:
Compose the custom image function with ImageAdjust:
Wavelet coefficients from stationary wavelet transform cannot be plotted using pyramid layout:
Pyramid layout cannot be constructed for inverse transformed wavelet coefficients:
Related Guides
History
Text
Wolfram Research (2010), WaveletImagePlot, Wolfram Language function, https://reference.wolfram.com/language/ref/WaveletImagePlot.html.
CMS
Wolfram Language. 2010. "WaveletImagePlot." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/WaveletImagePlot.html.
APA
Wolfram Language. (2010). WaveletImagePlot. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/WaveletImagePlot.html
BibTeX
@misc{reference.wolfram_2025_waveletimageplot, author="Wolfram Research", title="{WaveletImagePlot}", year="2010", howpublished="\url{https://reference.wolfram.com/language/ref/WaveletImagePlot.html}", note=[Accessed: 28-October-2025]}
BibLaTeX
@online{reference.wolfram_2025_waveletimageplot, organization={Wolfram Research}, title={WaveletImagePlot}, year={2010}, url={https://reference.wolfram.com/language/ref/WaveletImagePlot.html}, note=[Accessed: 28-October-2025]}