CoefficientArrays

CoefficientArrays[polys,vars]

gives the arrays of coefficients of the variables vars in the polynomials polys.

Details and Options

  • CoefficientArrays gives a list containing SparseArray objects, which can be converted to ordinary arrays using Normal.
  • If CoefficientArrays[polys,vars] gives {m0,m1,m2,…}, then polys can be reconstructed as m0+m1.vars+m2.vars.vars+…. »
  • Any element of polys of the form lhs==rhs is taken to correspond to the polynomial lhs-rhs. »
  • CoefficientArrays[polys,{form1,form2,…}] takes all expressions in polys that match any of the formi to be variables.
  • CoefficientArrays[polys] is equivalent to CoefficientArrays[polys,Variables[polys]].
  • The length of the list CoefficientArrays[polys,vars] is one more than the total degree of polys.
  • The mi are sparse arrays with ranks .
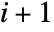  • The first element m0 has the same length as the list polys.
  • If polys is a single polynomial rather than a list, m0 is also not a list.
  • For linear equations, the solution to Thread[polys==0] is given by LinearSolve[m1,-m0].
  • For nonlinear equations, the mi are not unique. CoefficientArrays by default assigns nonzero coefficients only to monomials where the variables appear in the same order as vars.
  • CoefficientArrays[polys,vars,"Symmetric"->True] makes all the mi symmetric in all their indices. The resulting arrays will generally be less sparse.

Examples

open allclose all

Basic Examples  (1)

Scope  (4)

Linear polynomial:

List of linear polynomials:

Nonlinear polynomials:

Polynomial equations:

Options  (1)

"Symmetric"  (1)

The default coefficient of a quadratic form corresponding to y x is zero:

With "Symmetric"->True, CoefficientArrays gives symmetric arrays:

Applications  (1)

Convert linear equations to arrays suitable for LinearSolve:

Properties & Relations  (3)

The polynomial can be reconstructed by using Dot (.):

The symmetric coefficient arrays correspond to vector derivatives at the origin:

CoefficientArrays gives a list of arrays of polynomial coefficients ordered by total degrees:

CoefficientList gives a tensor of the coefficients:

Use Coefficient to find the coefficient of :

In ca the position of this coefficient is a+b+1 followed by a 1s and b 2s (1 and 2 indicate the first and second variables):

In cl the coefficient of x^a y^b is the element at position {a+1,b+1}:

Wolfram Research (2003), CoefficientArrays, Wolfram Language function, https://reference.wolfram.com/language/ref/CoefficientArrays.html.

Text

Wolfram Research (2003), CoefficientArrays, Wolfram Language function, https://reference.wolfram.com/language/ref/CoefficientArrays.html.

CMS

Wolfram Language. 2003. "CoefficientArrays." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/CoefficientArrays.html.

APA

Wolfram Language. (2003). CoefficientArrays. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/CoefficientArrays.html

BibTeX

@misc{reference.wolfram_2024_coefficientarrays, author="Wolfram Research", title="{CoefficientArrays}", year="2003", howpublished="\url{https://reference.wolfram.com/language/ref/CoefficientArrays.html}", note=[Accessed: 21-November-2024 ]}

BibLaTeX

@online{reference.wolfram_2024_coefficientarrays, organization={Wolfram Research}, title={CoefficientArrays}, year={2003}, url={https://reference.wolfram.com/language/ref/CoefficientArrays.html}, note=[Accessed: 21-November-2024 ]}