InverseBilateralLaplaceTransform[expr,s,t]
gives the inverse bilateral Laplace transform of expr.
InverseBilateralLaplaceTransform[expr,{s1,s2,…,sn},{t1,t2,…,tn}]
gives the multidimensional inverse bilateral Laplace transform of expr.


InverseBilateralLaplaceTransform
InverseBilateralLaplaceTransform[expr,s,t]
gives the inverse bilateral Laplace transform of expr.
InverseBilateralLaplaceTransform[expr,{s1,s2,…,sn},{t1,t2,…,tn}]
gives the multidimensional inverse bilateral Laplace transform of expr.
Details and Options

- The inverse bilateral Laplace transform of a function
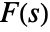 is defined to be
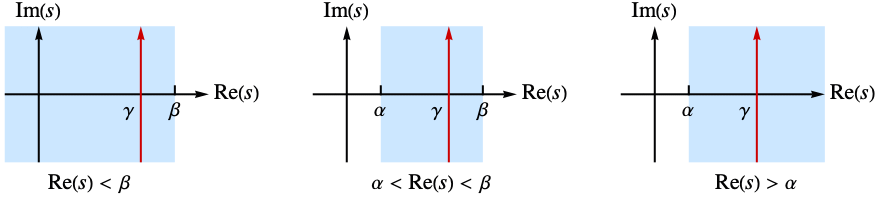
is defined to be 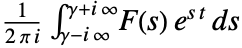 , where the integration is along a vertical line
, where the integration is along a vertical line 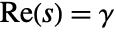 , lying in a strip
, lying in a strip 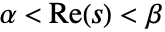 in which the function
in which the function  is holomorphic. In some cases, the strip of analyticity may extend to a half-plane.
is holomorphic. In some cases, the strip of analyticity may extend to a half-plane. - The multidimensional inverse bilateral Laplace transform of a function
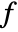 is given by a contour integral of the form
is given by a contour integral of the form 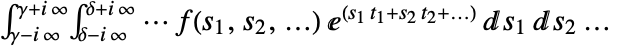 .
. - The integral is computed using numerical methods if the third argument,
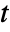 , is given a numerical value.
, is given a numerical value. - The following options can be given:
-
AccuracyGoal Automatic digits of absolute accuracy sought Assumptions $Assumptions assumptions to make about parameters GenerateConditions False whether to generate answers that involve conditions on parameters Method Automatic method to use PerformanceGoal $PerformanceGoal aspects of performance to optimize PrecisionGoal Automatic digits of precision sought WorkingPrecision Automatic the precision used in internal computations
Examples
open all close allBasic Examples (2)
Scope (13)
Inverse bilateral Laplace transform of rational function with two real poles:
Rational function with two real and two complex poles:
The following function has two real and four complex poles:
Inverse bilateral Laplace transform of a product of rational and exponential functions:
A rational function with different strips of convergences has different inverse bilateral Laplace transforms:
Rational function whose region of convergence is in the left half-plane:
Function with region of convergence in the right half-plane:
The inverse bilateral Laplace transform of the following rational function is a decaying sinusoidal wave:
Inverse bilateral Laplace transform of a function that is analytic in the whole complex plane:
Inverse bilateral Laplace transform leading to a Gaussian function:
Inverse bilateral Laplace transform of a constant is a Dirac delta function:
Evaluate the inverse bilateral Laplace transform at a single point:
Inverse bilateral Laplace transform at a single point for an analytic function:
Options (3)
Assumptions (3)
Specify the range for a parameter using Assumptions:
Use Assumptions to place a pole outside the strip of convergence:
Use Assumptions to restrict the right end of the convergence strip in the left half-plane:
Properties & Relations (1)
InverseBilateralLaplaceTransform and BilateralLaplaceTransform are mutual inverses:
Related Guides
History
Text
Wolfram Research (2021), InverseBilateralLaplaceTransform, Wolfram Language function, https://reference.wolfram.com/language/ref/InverseBilateralLaplaceTransform.html.
CMS
Wolfram Language. 2021. "InverseBilateralLaplaceTransform." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/InverseBilateralLaplaceTransform.html.
APA
Wolfram Language. (2021). InverseBilateralLaplaceTransform. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/InverseBilateralLaplaceTransform.html
BibTeX
@misc{reference.wolfram_2025_inversebilaterallaplacetransform, author="Wolfram Research", title="{InverseBilateralLaplaceTransform}", year="2021", howpublished="\url{https://reference.wolfram.com/language/ref/InverseBilateralLaplaceTransform.html}", note=[Accessed: 22-October-2025]}
BibLaTeX
@online{reference.wolfram_2025_inversebilaterallaplacetransform, organization={Wolfram Research}, title={InverseBilateralLaplaceTransform}, year={2021}, url={https://reference.wolfram.com/language/ref/InverseBilateralLaplaceTransform.html}, note=[Accessed: 22-October-2025]}