InverseLaplaceTransform

InverseLaplaceTransform[F[s],s,t]

gives the symbolic inverse Laplace transform of F[s] in the variable s as f[t] in the variable t.

InverseLaplaceTransform[F[s],s,]

gives the numeric inverse Laplace transform at the numerical value .

InverseLaplaceTransform[F[s1,…,sn],{s1,s2,…},{t1,t2,…}]

gives the multidimensional inverse Laplace transform of F[s1,…,sn].

Details and Options

  • Laplace transforms are typically used to transform differential and partial differential equations to algebraic equations, solve and then inverse transform back to a solution.
  • Laplace transforms are also extensively used in control theory and signal processing as a way to represent and manipulate linear systems in the form of transfer functions and transfer matrices. The Laplace transform and its inverse are then a way to transform between the time domain and frequency domain.
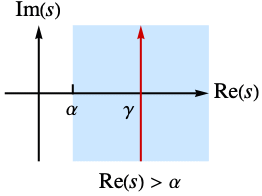
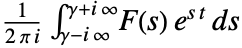  • The inverse Laplace transform of a function is defined to be , where γ is an arbitrary positive constant chosen so that the contour of integration lies to the right of all singularities in .
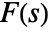  • The multidimensional inverse Laplace transform of a function is given by a contour integral of the form .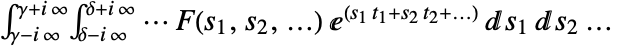
  • The integral is computed using numerical methods if the third argument 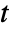is given a numerical value. The available method settings include "Crump", "Durbin", "Papoulis", "Piessens", "Stehfest", "Talbot" and "Weeks".
  • The asymptotic inverse Laplace transform can be computed using Asymptotic.
  • The following options can be given:
  • AccuracyGoalAutomaticdigits of absolute accuracy sought
    Assumptions$Assumptionsassumptions to make about parameters
    GenerateConditions Falsewhether to generate answers that involve conditions on parameters
    Method Automaticmethod to use
    PerformanceGoal$PerformanceGoalaspects of performance to optimize
    PrecisionGoalAutomaticdigits of precision sought
    WorkingPrecisionAutomaticthe precision used in internal computations
  • In TraditionalForm, InverseLaplaceTransform is output using ℒ-1. »

Examples

open allclose all

Basic Examples  (4)

Compute the inverse Laplace transform of a function:

Inverse Laplace transform of a function with parameters:

Plot the result:

Compute a numerical inverse Laplace transform:

Inverse Laplace transform of a bivariate function:

Scope  (56)

Basic Uses  (3)

Compute the inverse Laplace transform of a function for a symbolic parameter t:

Use a numerical value for the parameter:

TraditionalForm formatting:

Rational Functions  (5)

Reciprocal of a linear function:

Reciprocal of a quadratic function:

Functions whose inverses are trigonometric functions:

Functions whose inverses are hyperbolic functions:

More examples of rational functions:

Elementary Functions  (5)

Function involving a square root:

Function involving exponential and square root functions:

Other algebraic functions:

Inverse Laplace transform leading to a Bessel function:

Inverse of an arctangent function:

Logarithmic Functions  (4)

Inverse Laplace transform of a logarithmic function:

Logarithm of a rational function:

Plot the result:

Product of a logarithm and a power function:

Function involving the square of a logarithm:

Special Functions  (12)

Function involving BesselK:

Ratio of an incomplete gamma function and a power function:

Inverse of a polygamma function:

Inverse of a function involving an error function:

Error function composed with a square root:

Inverse transforms of the LegendreP and LegendreQ functions:

Complex plots of the Legendre functions:

Product of BesselI and Exp:

ComplexPlot of the frequency-domain function:

Product of BesselJ and Gamma functions:

ComplexPlot of the frequency-domain function:

Composition of exponential integral and square root functions:

Product of two ParabolicCylinderD functions:

Inverse transforms involving elliptic integral functions:

Inverse transform of EllipticTheta:

Piecewise Functions  (5)

The inverse of the following function is a piecewise function:

Plot the inverse:

Inverse of an exponential function leading to a box function:

Inverse of an exponential function leading to a piecewise trigonometric function:

Inverse leading to a staircase function:

More involved staircase function:

Periodic Functions  (4)

The inverse of the following function is periodic with respect to t:

Activate the sum:

Plot to verify that the result is a square wave:

Function whose inverse is the absolute value of the sine function:

Activate and plot the result:

Function whose inverse is a trapezoidal wave:

A more involved piecewise example:

Generalized Functions  (3)

Inverse of an exponential function is the Dirac function:

Inverse of the product of an exponential function and a power of s is a derivative of the Dirac function:

The inverse of the hyperbolic secant is a series of Dirac functions:

Hyperbolic cotangent:

Multivariate Functions  (8)

Inverse Laplace transform of a bivariate rational function:

Verify the result:

Rational function that is separable with respect to p and q:

Rational function that is not separable with respect to p and q:

Rational function whose inverse is related to BesselJ:

Inverse Laplace transform of a bivariate function involving a radical:

Inverse Laplace transform of a multivariate function in three variables:

Rational function:

Function involving a logarithm:

Numerical Inversion  (4)

Calculate the inverse Laplace transform at a single point:

Alternatively, calculate the inverse Laplace transform symbolically:

Then evaluate it for a specific value of t:

Plot the inverse Laplace transform numerically and compare it with the exact result:

Function whose inverse is a piecewise function with respect to t:

Function whose inverse is a periodic function with respect to t:

Fractional Calculus  (3)

ComplexPlot of an algebraic function in the -domain:

Inverse Laplace transform of this algebraic function:

Laplace transform to the -domain:

Inverse Laplace transform of the algebraic function:

Plot in time domain:

Laplace transform to the -domain:

Inverse Laplace transform of the algebraic function involving parameters:

Laplace transform to the -domain:

Options  (3)

GenerateConditions  (1)

By default, InverseLaplaceTransform assumes that the result is defined for non-negative t:

Use GenerateConditions to obtain the range of validity for the result:

Method  (1)

Use the default method for numerical evaluation:

Use Method to obtain the result from different methods:

Working Precision  (1)

Use WorkingPrecision to obtain a result with arbitrary precision:

Applications  (5)

Compute the step response to the linear system with transfer function :

Solve a differential equation using Laplace transforms:

Solve for the Laplace transform:

Find the inverse transform:

Apply initial conditions:

Find the solution directly using DSolve:

Solve a fractional-order differential equation of order 3/2:

Solve for the Laplace transform:

Find the inverse transform:

Plot the solution:

Find the solution directly using DSolve:

Solve a fractional-order differential equation of order 21/10:

Solve for the Laplace transform:

Find the inverse transform:

Plot the solution:

Find the solution directly using DSolve:

Solve a system of fractional DEs using LaplaceTransform:

Find the inverse transform:

Properties & Relations  (2)

Use Asymptotic to compute an asymptotic approximation:

InverseLaplaceTransform and LaplaceTransform are mutual inverses:

Neat Examples  (2)

InverseLaplaceTransform of a MeijerG function:

Create a table of basic inverse Laplace transforms:

Wolfram Research (1999), InverseLaplaceTransform, Wolfram Language function, https://reference.wolfram.com/language/ref/InverseLaplaceTransform.html (updated 2023).

Text

Wolfram Research (1999), InverseLaplaceTransform, Wolfram Language function, https://reference.wolfram.com/language/ref/InverseLaplaceTransform.html (updated 2023).

CMS

Wolfram Language. 1999. "InverseLaplaceTransform." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2023. https://reference.wolfram.com/language/ref/InverseLaplaceTransform.html.

APA

Wolfram Language. (1999). InverseLaplaceTransform. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/InverseLaplaceTransform.html

BibTeX

@misc{reference.wolfram_2023_inverselaplacetransform, author="Wolfram Research", title="{InverseLaplaceTransform}", year="2023", howpublished="\url{https://reference.wolfram.com/language/ref/InverseLaplaceTransform.html}", note=[Accessed: 30-November-2023 ]}

BibLaTeX

@online{reference.wolfram_2023_inverselaplacetransform, organization={Wolfram Research}, title={InverseLaplaceTransform}, year={2023}, url={https://reference.wolfram.com/language/ref/InverseLaplaceTransform.html}, note=[Accessed: 30-November-2023 ]}