SolarEclipse

SolarEclipse[]

gives the time of the next solar eclipse.

SolarEclipse[datespec]

gives the time for the next solar eclipse after the specified date.

SolarEclipse[propertyspec]

gives the specified property value for the next solar eclipse.

SolarEclipse[datespec,propertyspec]

gives the specified property value for the next solar eclipse after the specified date.

Details and Options

  • SolarEclipse[] makes use of $TimeZone to determine your time zone.
  • datespec can be a DateObject expression, a TimeObject expression, a date string or a {y,m,d,h,m,s} date list.
  • datespec is assumed to be in $TimeZone, unless it is a DateObject or TimeObject expression with an explicit TimeZone option value.
  • If datespec is a list of dates, then the results will contain EventSeries or TimeSeries objects.
  • datespec can be specified as {start,end,increment} for compatibility with DateRange specifications.
  • datespec can be specified as {start,end,All} to obtain a list of the property values for all unique eclipses in the specified range.
  • SolarEclipse[TimeZone->z] gives the date of the next solar eclipse with date and time inferred for time zone z by assuming that your computer is set for the time zone specified by $TimeZone.
  • SolarEclipse["MaximumEclipseDate"] is equivalent to SolarEclipse[].
  • SolarEclipse can accept the following values for propertyspec:
  • "GraphicsData"graphics directives for all phases of the eclipse
    "MaximumEclipseDate"date of the maximum of the eclipse
    "PartialPhasePolygon"polygon representing the partial phase of the eclipse
    "TotalPhaseEndDate"date of the end of the total phase of the eclipse
    "TotalPhaseStartDate"date of the start of the total phase of the eclipse
    "TotalPhaseCenterLine"line representing the central path of the eclipse
    "TotalPhasePolygon"polygon representing the total phase of the eclipse
    "Type"type of the eclipse
  • SolarEclipse can accept the following options:
  • TimeDirection1whether to return the next or last solar eclipse
    EclipseTypeAutomaticused to specify the type of solar eclipse
    TimeZone$TimeZonetime zone to be used for dates
  • Possible settings for TimeDirection include:
  • 1returns the next solar eclipse from the specified date
    -1returns the last solar eclipse from the specified date
  • Possible settings for EclipseType include:
  • Automaticreturns the next eclipse of any type
    "Annular"returns the next annular eclipse
    "Hybrid"returns the next hybrid eclipse
    "Partial"returns the next partial eclipse
    "Total"returns the next total eclipse

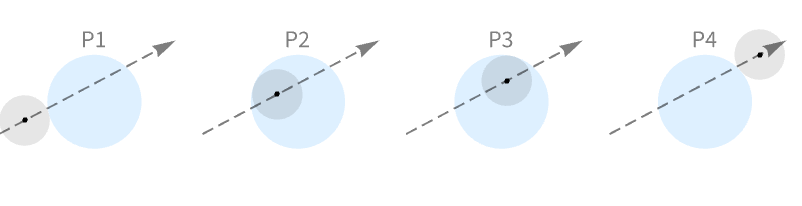
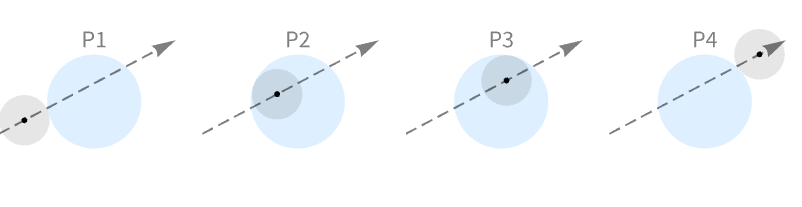
Examples

open allclose all

Basic Examples  (3)

Find the date of the next solar eclipse:

Find the date of the next solar eclipse after a specified date:

Find the type of the next solar eclipse:

Scope  (12)

Dates  (4)

Dates can be specified as a DateObject:

Dates can be specified as a date string:

Generate the next solar eclipse for a range of dates:

Generate a list of the dates of unique solar eclipses for a range of dates:

Properties  (8)

Generate a map showing the regions of partiality and totality for the first total solar eclipse in 2017:

Determine the starting date for the first solar eclipse in 2017:

Generate a map showing the region of partiality for the first total solar eclipse in 2017:

Determine the ending date for the first solar eclipse in 2017:

Determine the starting date for the first total solar eclipse in 2017:

Generate a map showing the region of totality for the first total solar eclipse in 2017:

Generate a map showing the region of totality for the first total solar eclipse in 2017:

Determine the type of the first solar eclipse in 2017:

Possible Issues  (2)

Short date specifications are automatically padded; the following example is understood to mean the first solar eclipse after midnight on January 1, 2017:

The following expanded form gives the same result:

Neat Examples  (1)

Generate a map showing the paths of all total solar eclipses between 2010 and 2020:

Wolfram Research (2014), SolarEclipse, Wolfram Language function, https://reference.wolfram.com/language/ref/SolarEclipse.html.

Text

Wolfram Research (2014), SolarEclipse, Wolfram Language function, https://reference.wolfram.com/language/ref/SolarEclipse.html.

CMS

Wolfram Language. 2014. "SolarEclipse." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/SolarEclipse.html.

APA

Wolfram Language. (2014). SolarEclipse. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/SolarEclipse.html

BibTeX

@misc{reference.wolfram_2022_solareclipse, author="Wolfram Research", title="{SolarEclipse}", year="2014", howpublished="\url{https://reference.wolfram.com/language/ref/SolarEclipse.html}", note=[Accessed: 01-April-2023 ]}

BibLaTeX

@online{reference.wolfram_2022_solareclipse, organization={Wolfram Research}, title={SolarEclipse}, year={2014}, url={https://reference.wolfram.com/language/ref/SolarEclipse.html}, note=[Accessed: 01-April-2023 ]}