GraphJoin[g1,g2]
gives the graph join of the graphs g1 and g2.


GraphJoin
GraphJoin[g1,g2]
gives the graph join of the graphs g1 and g2.
Details and Options

- GraphJoin is also known as complete union.
- GraphJoin is typically used to produce new graphs with particular reachable subgraphs.
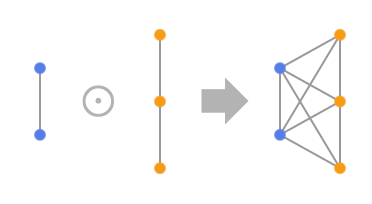
- GraphJoin[g1,g2] gives a graph obtained from g1 and g2 by joining each vertex of g1 to all vertices of g2.
- GraphJoin[g1,g2] returns GraphDisjointUnion[g1,g2] together with all edges between each vertex of IndexGraph[g1] and each vertex of IndexGraph[g2,n+1], where n is the VertexCount for g1.
- GraphJoin takes the same options as Graph.
-
AlignmentPoint Center the default point in the graphic to align with AnnotationRules {} annotations for graph, edges and vertices AspectRatio Automatic ratio of height to width Axes False whether to draw axes AxesLabel None axes labels AxesOrigin Automatic where axes should cross AxesStyle {} style specifications for the axes Background None background color for the plot BaselinePosition Automatic how to align with a surrounding text baseline BaseStyle {} base style specifications for the graphic ContentSelectable Automatic whether to allow contents to be selected CoordinatesToolOptions Automatic detailed behavior of the coordinates tool DirectedEdges Automatic whether to interpret Rule as DirectedEdge EdgeLabels None labels and label placements for edges EdgeLabelStyle Automatic style to use for edge labels EdgeShapeFunction Automatic generate graphic shapes for edges EdgeStyle Automatic style used for edges EdgeWeight Automatic weights for edges Epilog {} primitives rendered after the main plot FormatType TraditionalForm the default format type for text Frame False whether to put a frame around the plot FrameLabel None frame labels FrameStyle {} style specifications for the frame FrameTicks Automatic frame ticks FrameTicksStyle {} style specifications for frame ticks GraphHighlight {} graph elements to highlight GraphHighlightStyle Automatic style for highlight GraphLayout Automatic how to lay out vertices and edges GridLines None grid lines to draw GridLinesStyle {} style specifications for grid lines ImageMargins 0. the margins to leave around the graphic ImagePadding All what extra padding to allow for labels etc. ImageSize Automatic the absolute size at which to render the graphic LabelStyle {} style specifications for labels Method Automatic details of graphics methods to use PerformanceGoal Automatic aspects of performance to try to optimize PlotLabel None an overall label for the plot PlotRange All range of values to include PlotRangeClipping False whether to clip at the plot range PlotRangePadding Automatic how much to pad the range of values PlotRegion Automatic the final display region to be filled PlotTheme $PlotTheme overall theme for the graph PreserveImageOptions Automatic whether to preserve image options when displaying new versions of the same graphic Prolog {} primitives rendered before the main plot RotateLabel True whether to rotate y labels on the frame Ticks Automatic axes ticks TicksStyle {} style specifications for axes ticks VertexCoordinates Automatic coordinates for vertices VertexLabels None labels and placements for vertices VertexLabelStyle Automatic style to use for vertex labels VertexShape Automatic graphic shape for vertices VertexShapeFunction Automatic generate graphic shapes for vertices VertexSize Medium size of vertices VertexStyle Automatic styles for vertices VertexWeight Automatic weights for vertices
List of all options


Examples
open all close allBasic Examples (3)
Scope (29)
Properties & Relations (4)
Joining the adjacency matrix of two graphs on diagonal and others are ones gets the adjacency matrix of GraphJoin:
The join of a single vertex ![]() to an empty graph gets a star graph:
to an empty graph gets a star graph:
The join of a single vertex ![]() to a cycle graph
to a cycle graph ![]() gets a wheel graph
gets a wheel graph ![]() :
:
The join of empty graphs on ![]() ,
, ![]() , ... nodes gets a complete
, ... nodes gets a complete ![]() -partite graph
-partite graph ![]() :
:
Related Guides
History
Text
Wolfram Research (2022), GraphJoin, Wolfram Language function, https://reference.wolfram.com/language/ref/GraphJoin.html.
CMS
Wolfram Language. 2022. "GraphJoin." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/GraphJoin.html.
APA
Wolfram Language. (2022). GraphJoin. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/GraphJoin.html
BibTeX
@misc{reference.wolfram_2025_graphjoin, author="Wolfram Research", title="{GraphJoin}", year="2022", howpublished="\url{https://reference.wolfram.com/language/ref/GraphJoin.html}", note=[Accessed: 24-October-2025]}
BibLaTeX
@online{reference.wolfram_2025_graphjoin, organization={Wolfram Research}, title={GraphJoin}, year={2022}, url={https://reference.wolfram.com/language/ref/GraphJoin.html}, note=[Accessed: 24-October-2025]}