LineIntegrate

LineIntegrate[f,{x,y,…}∈curve]

computes the scalar line integral of the function f[x,y,…] over the curve.

LineIntegrate[{p,q,…},{x,y,…}∈curve]

computes the vector line integral of the vector function {p[x,y,…],q[x,y,…],…}.

Details and Options

  • Line integrals are also known as curve integrals and work integrals.
  • Scalar line integrals integrate scalar function along a curve. They typically compute things like length, mass and charge for a curve.
  • Vector line integrals are used to compute the work done by a vector function as it moves along a curve in the direction of its tangent. Typical vector functions include a force field, electric field and fluid velocity field.
  • The scalar line integral of the function f along a curve 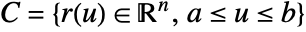is given by:
  • where TemplateBox[{{{r, ^, {(, ', )}}, (, u, )}}, Norm] is the measure of a parametric curve segment.
  • The scalar line integral is independent of the parametrization and orientation of the curve. Any one dimensional RegionQ object can be used as curve.
  • The vector line integral of the function F along a curve 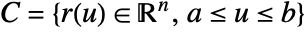is given by:
  • where 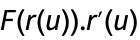F(r(u)).r^(')(u) is projection of the vector function 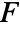onto the tangent direction so only the component in the tangent direction gets integrated.
  • The vector line integral is independent of the parametrization of the curve, but it does depend on the orientation of the curve.
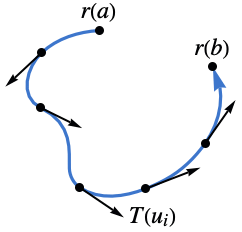
  • The orientation for a curve is given by a tangent vector field 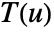over the curve.
  • For a parametric curve ParametricRegion[{r1[u],…,rn[u]},…], the tangent vector field 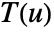is taken to be ∂ur[u].
  • Special curves in with their assumed tangent orientation include:
  • Line[{p1,p2,…}]the orientation follows the points in the order they are given from p1 to p2 etc.
    HalfLine[{p1,p2}]
    HalfLine[p,v]    		the orientation is from p1 to p2 or in the v direction
    InfiniteLine[{p1,p2}]
    InfiniteLine[p,v]    		the orientation is from p1 to p2 or in the v direction
    Circle[p,r]the orientation is counterclockwise
  • Special curves in with their assumed tangent orientation include:
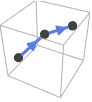
  • Line[{p1,p2,…}]the orientation follows the points in the order they are given from p1 to p2 etc.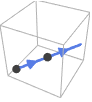
    HalfLine[{p1,p2}]
    HalfLine[p,v]    		the orientation is from p1 to p2 or in the v direction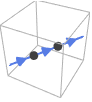
    InfiniteLine[{p1,p2}]
    InfiniteLine[p,v]    		the orientation is from p1 to p2 or in the v direction
  • Special curves in 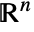with their assumed tangent orientation include:
  • Line[{p1,p2,…}]the orientation follows the points in the order they are given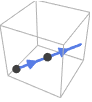
    HalfLine[{p1,p2}]
    HalfLine[p,v]    		the orientation is from p1 to p2 the orientation is given by v
    InfiniteLine[{p1,p2}]
    InfiniteLine[p,v]    		the orientation is from p1 to p2 the orientation is given by v
  • The following options can be given:
  • Assumptions $Assumptionsassumptions to make about parameters
    Direction Automaticorientation of the curve
    GenerateConditions Automaticwhether to generate answers that involve conditions on parameters
    WorkingPrecision Automaticthe precision used in internal computations
  • LineIntegrate uses a combination of symbolic and numerical methods when the input involves inexact quantities.

Examples

open allclose all

Basic Examples  (6)

Line integral of a scalar field over a circle:

Line integral of a vector field over a line segment:

Line integral of a vector field over a space curve:

Line integral of a scalar field in two dimensions:

Curve over which to integrate:

A contour plot of and the curve:

The line integral:

Line integral of a vector field in two dimensions:

The vector field and the integration path:

The line integral:

Line integral of a vector field in three dimensions:

Scope  (32)

Basic Uses  (4)

Line integral of a scalar field:

Line integral of a vector field in three dimensions:

LineIntegrate works with many special curves:

Line integral over a parametric curve:

Scalar Functions  (11)

Line integral of a scalar field over a curve:

Contour plot of and the curve:

Line integral:

Line integral of a scalar field over an arc of a circle:

Line integral of a scalar field over a parametric curve:

Contour plot of the function and the curve:

Line integral of a scalar field over a circle:

Line integral of a scalar field over a space curve:

Line integral:

Line integral of a scalar field over the boundary of an annulus:

Contour plot of the function and the curve:

Line integral of a scalar field over a closed polygon:

Contour plot of the function and the curve:

Line integral of a scalar field over an elliptical path:

Contour plot of the function and the curve:

Line integral:

Line integral of a scalar field over a parametric curve:

Line integral:

Line integral of a scalar field over a circle:

Contour plot of and the curve:

Line integral:

Line integral of a scalar field over the boundary of a sector of a disk:

Contour plot of and the curve:

Line integral:

Vector Functions  (12)

Line integral of a vector field in three dimensions over a parametrized curve:

Visualization of the vector field and the curve:

Line integral:

Line integral of a vector field over a curve in two dimensions:

Line integral:

Line integral of a vector field over a circular arc:

Line integral of a vector field over a line segment:

Line integral of a vector field over a parametrized curve in three dimensions:

Line integral of a vector field over a curve:

Line integral of a vector field over an elliptical arc:

Line integral of a vector field over a parametric curve:

Line integral of a vector field over a parametric curve in three dimensions:

Line integral of a vector field over a parametrized curve:

Line integral of a vector field over an elliptical path:

Line integral of a vector field in higher dimensions:

Special Curves  (4)

Line integral over a circular arc:

Line integral of a vector field over the boundary of a circular sector of radius 1:

Line integral over a polygon:

Line integral over the boundary of an annulus:

Parametric Curves  (1)

Line integral of a vector field over a spiral in three dimensions:

Options  (5)

Assumptions  (1)

The option Assumptions can be used on parameters:

Direction  (1)

The default Direction for closed circular paths is counterclockwise:

Which is equivalent to:

The clockwise direction can be chosen using the option Direction:

GenerateConditions  (1)

LineIntegrate can work with symbolic parameters:

Suppress the conditions on the parameters:

WorkingPrecision  (2)

If a WorkingPrecision is specified, a numerical result is given:

The result has finite precision if the integrand has a finite precision:

Applications  (27)

College Calculus  (10)

Line integral of a function over a line segment:

Line integral of a vector field over a curve:

Mass of a thin circular wire of radius 1 with linear density :

Work done by the force field on a particle that moves along a line segment:

Line integral of a vector field along a path:

Line integral of a vector field along a curve:

Work done by the force as a particle moves along the curve :

Line integral of a vector field along the unit circle centered at the origin:

Line integral of a vector field along a circle of radius centered at the origin:

Numerical value of the line integral of a vector field over a path:

Lengths  (3)

Circumference of a circle:

Perimeter of a cardioid using a line integral:

The length can also be calculated with RegionMeasure:

Perimeter of an astroid:

Areas  (5)

Area of an ellipse with semiaxes and , calculated using a line integral:

Area of the right-hand loop of the lemniscate computed using a line integral:

Area of the epicycloid of parameters and :

Area of the cardioid using a line integral:

Area of an astroid using a line integral:

Work by a Force  (4)

Work done by the gravitational force as an object is moved on a straight line:

Work done by the electric force as a charge is moved from {1,1,0} to {2,2,0} in the electric field of a charged infinite wire of charge density :

Work done by an elastic force directed toward the origin as a quarter of an ellipse is traced:

Work of the electric force as a charge is moved along the axis from to infinity in the electric field of a charge :

Centroids  (2)

Mass of a closed semicircular wire of radius and linear density :

coordinate of the center of mass:

coordinate of the center of mass:

Moments of inertia of a helix-shaped wire of constant linear density :

About the axis:

About the axis:

About the axis:

Classical Theorems  (3)

A vector field is conservative if its line integral depends only on the values at the endpoints, not on the path:

The field is the gradient of a scalar function :

All gradients of scalar fields are conservative. For example, the line integral of over the curve is:

This is the same as the difference of the values of at the endpoints of the curve:

The line integral of the vector field over a closed curve is:

This can be related to a surface integral of over the region enclosed by the curve, where is defined as:

The line integral of a vector field along a closed line in three dimensions is:

This is equal to the surface integral of the Curl of on any surface having the curve as its boundary:

The surface integral across a different surface with the same boundary is the same:

Properties & Relations  (5)

Apply N[LineIntegrate[...]] to obtain a numerical solution if the symbolic calculation fails:

Find the center of mass of a triangular wire of unit linear density:

Find the total mass:

Find the component of the center of mass:

The center of mass can also be obtained using RegionCentroid:

Find the moment of inertia around the -axis of a circular wire of unit linear density centered at the origin in the - plane:

The answer can also be computed with MomentOfInertia:

Find the length of an epicycloid:

The same answer can be obtained using ArcLength:

Find the area of an ellipse:

The result can be obtained using RegionMeasure:

Neat Examples  (2)

Length of a catenary:

Integral of a vector field over a Clelia curve:

Wolfram Research (2023), LineIntegrate, Wolfram Language function, https://reference.wolfram.com/language/ref/LineIntegrate.html.

Text

Wolfram Research (2023), LineIntegrate, Wolfram Language function, https://reference.wolfram.com/language/ref/LineIntegrate.html.

CMS

Wolfram Language. 2023. "LineIntegrate." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/LineIntegrate.html.

APA

Wolfram Language. (2023). LineIntegrate. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/LineIntegrate.html

BibTeX

@misc{reference.wolfram_2024_lineintegrate, author="Wolfram Research", title="{LineIntegrate}", year="2023", howpublished="\url{https://reference.wolfram.com/language/ref/LineIntegrate.html}", note=[Accessed: 05-November-2024 ]}

BibLaTeX

@online{reference.wolfram_2024_lineintegrate, organization={Wolfram Research}, title={LineIntegrate}, year={2023}, url={https://reference.wolfram.com/language/ref/LineIntegrate.html}, note=[Accessed: 05-November-2024 ]}