NicholsPlot
✖
NicholsPlot
Details and Options
- NicholsPlot gives the magnitude versus phase plot of the transfer function of lsys.
- The system lsys can be TransferFunctionModel or StateSpaceModel, including descriptor and delay systems.
- For a system lsys with the corresponding transfer function
, the following expressions are plotted: -
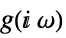
continuous-time system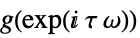 
discrete-time system with sample time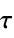 
- NicholsPlot treats the variable ω as local, effectively using Block.
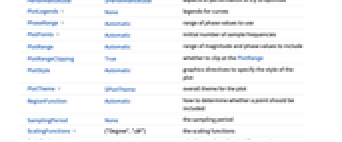
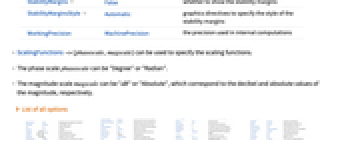
- NicholsPlot has the same options as Graphics, with the following changes and additions: [List of all options]
-
Axes True whether to draw axes ColorFunction Automatic how to apply coloring to the curve ColorFunctionScaling True whether to scale arguments to ColorFunction EvaluationMonitor None expression to evaluate at every evaluation Exclusions Automatic frequencies to exclude ExclusionsStyle None what to draw at excluded frequencies FeedbackType "Negative" the feedback type MaxRecursion Automatic the maximum number of recursive subdivisions allowed Mesh Automatic how many mesh divisions to draw MeshFunctions {#3&} how to determine the placement of mesh divisions MeshShading None how to shade regions between mesh points MeshStyle Automatic the style for mesh divisions NicholsGridLines None the Nichols grid lines to draw PerformanceGoal $PerformanceGoal aspects of performance to try to optimize PlotLegends None legends for curves PhaseRange Automatic range of phase values to use PlotPoints Automatic iniitial number of sample frequencies PlotRange Automatic range of magnitude and phase values to include PlotRangeClipping True whether to clip at the PlotRange PlotStyle Automatic graphics directives to specify the style of the plot PlotTheme $PlotTheme overall theme for the plot RegionFunction Automatic how to determine whether a point should be included SamplingPeriod None the sampling period ScalingFunctions {"Degree","dB"} the scaling functions StabilityMargins False whether to show the stability margins StabilityMarginsStyle Automatic graphics directives to specify the style of the stability margins WorkingPrecision MachinePrecision the precision used in internal computations - ScalingFunctions->{phasescale,magscale} can be used to specify the scaling functions.
- The phase scale phasescale can be "Degree" or "Radian".
- The magnitude scale magscale can be "dB" or "Absolute", which correspond to the decibel and absolute values of the magnitude, respectively.
-
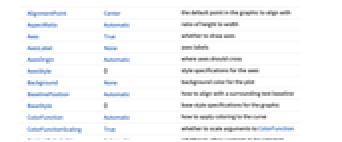
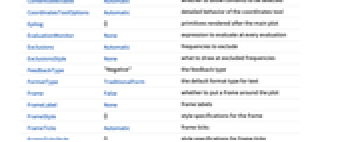
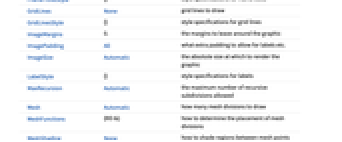
AlignmentPoint Center the default point in the graphic to align with AspectRatio Automatic ratio of height to width Axes True whether to draw axes AxesLabel None axes labels AxesOrigin Automatic where axes should cross AxesStyle {} style specifications for the axes Background None background color for the plot BaselinePosition Automatic how to align with a surrounding text baseline BaseStyle {} base style specifications for the graphic ColorFunction Automatic how to apply coloring to the curve ColorFunctionScaling True whether to scale arguments to ColorFunction ContentSelectable Automatic whether to allow contents to be selected CoordinatesToolOptions Automatic detailed behavior of the coordinates tool Epilog {} primitives rendered after the main plot EvaluationMonitor None expression to evaluate at every evaluation Exclusions Automatic frequencies to exclude ExclusionsStyle None what to draw at excluded frequencies FeedbackType "Negative" the feedback type FormatType TraditionalForm the default format type for text Frame False whether to put a frame around the plot FrameLabel None frame labels FrameStyle {} style specifications for the frame FrameTicks Automatic frame ticks FrameTicksStyle {} style specifications for frame ticks GridLines None grid lines to draw GridLinesStyle {} style specifications for grid lines ImageMargins 0. the margins to leave around the graphic ImagePadding All what extra padding to allow for labels etc. ImageSize Automatic the absolute size at which to render the graphic LabelStyle {} style specifications for labels MaxRecursion Automatic the maximum number of recursive subdivisions allowed Mesh Automatic how many mesh divisions to draw MeshFunctions {#3&} how to determine the placement of mesh divisions MeshShading None how to shade regions between mesh points MeshStyle Automatic the style for mesh divisions Method Automatic details of graphics methods to use NicholsGridLines None the Nichols grid lines to draw PerformanceGoal $PerformanceGoal aspects of performance to try to optimize PhaseRange Automatic range of phase values to use PlotLabel None an overall label for the plot PlotLegends None legends for curves PlotPoints Automatic iniitial number of sample frequencies PlotRange Automatic range of magnitude and phase values to include PlotRangeClipping True whether to clip at the PlotRange PlotRangePadding Automatic how much to pad the range of values PlotRegion Automatic the final display region to be filled PlotStyle Automatic graphics directives to specify the style of the plot PlotTheme $PlotTheme overall theme for the plot PreserveImageOptions Automatic whether to preserve image options when displaying new versions of the same graphic Prolog {} primitives rendered before the main plot RegionFunction Automatic how to determine whether a point should be included RotateLabel True whether to rotate y labels on the frame SamplingPeriod None the sampling period ScalingFunctions {"Degree","dB"} the scaling functions StabilityMargins False whether to show the stability margins StabilityMarginsStyle Automatic graphics directives to specify the style of the stability margins Ticks Automatic axes ticks TicksStyle {} style specifications for axes ticks WorkingPrecision MachinePrecision the precision used in internal computations
List of all options
Examples
open allclose allBasic Examples (4)Summary of the most common use cases
Nichols plot of a transfer-function model:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-0xq4s
Out[1]=1
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-qgf9hw
Out[1]=1
Nichols plot of a discrete-time system:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-iel4se
Out[1]=1
Use legends for multiple systems:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-62p7si
Out[1]=1
Scope (8)Survey of the scope of standard use cases
The Nichols plot of a continuous-time system:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-dr1dai
Out[1]=1
The Nichols plot of a discrete-time system:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-qugeo6
Out[1]=1
The system can also be specified as an expression:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-n8rham
Out[1]=1
A discrete-time system with sampling period 1, specified as an expression:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-305n7x
Out[1]=1
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-lnklfv
Out[1]=1
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-62iaf9
Out[1]=1
The Nichols plot of a state-space model:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-45m3fg
Out[1]=1
A system specified as a sinusoidal transfer function:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-qlg90z
Out[1]=1
Generalizations & Extensions (1)Generalized and extended use cases
NicholsPlot[TransferFunctionModel[g,var]] is equivalent to NicholsPlot[g]:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-ghj59y
In[2]:=2
✖
https://wolfram.com/xid/0k767dltnsyqq-kzc566
Out[2]=2
Options (28)Common values & functionality for each option
AspectRatio (1)
ColorFunction (3)
Color the curve by scaled frequency values:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-ybm81r
Out[1]=1
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-730rt2
Out[1]=1
Use red when the sensitivity function is less than 1, and black otherwise:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-mrwtk4
Out[1]=1
ColorFunctionScaling (1)
Scale the frequency to be between 0 and 1:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-7bk4cg
Out[1]=1
ColorFunctionScaling->False uses absolute values:
In[2]:=2
✖
https://wolfram.com/xid/0k767dltnsyqq-7ztco2
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0k767dltnsyqq-8fa48
Out[3]=3
CoordinatesToolOptions (1)
Exclusions (1)
Mesh (4)
Show equally spaced frequency locations:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-te3nse
Out[1]=1
Show the coordinates at 1 radian per time unit:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-cv64nn
Out[1]=1
Show the coordinates at several frequencies:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-squdeb
Out[1]=1
Specify the graphics directives:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-u4nbvg
Out[1]=1
MeshFunctions (1)
MeshStyle (1)
NicholsGridLines (2)
PhaseRange (1)
PlotLegends (4)
Use automatic legends for multiple systems:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-x35f4u
Out[1]=1
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-s5n7t3
Out[1]=1
Use LineLegend to add the label for overall legend:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-2x6exd
Out[1]=1
Place the legend above the plot:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-x3yutu
Out[1]=1
PlotPoints (1)
PlotTheme (2)
ScalingFunctions (1)
StabilityMargins (3)
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-iooufm
Out[1]=1
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-oaaexb
Out[1]=1
Only margins and crossover frequencies with numerical values are shown:
In[1]:=1
✖
https://wolfram.com/xid/0k767dltnsyqq-p2yc79
In[2]:=2
✖
https://wolfram.com/xid/0k767dltnsyqq-c8q2ur
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0k767dltnsyqq-llfi22
Out[3]=3
Wolfram Research (2010), NicholsPlot, Wolfram Language function, https://reference.wolfram.com/language/ref/NicholsPlot.html (updated 2014).
✖
Wolfram Research (2010), NicholsPlot, Wolfram Language function, https://reference.wolfram.com/language/ref/NicholsPlot.html (updated 2014).Text
Wolfram Research (2010), NicholsPlot, Wolfram Language function, https://reference.wolfram.com/language/ref/NicholsPlot.html (updated 2014).
✖
Wolfram Research (2010), NicholsPlot, Wolfram Language function, https://reference.wolfram.com/language/ref/NicholsPlot.html (updated 2014).CMS
Wolfram Language. 2010. "NicholsPlot." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2014. https://reference.wolfram.com/language/ref/NicholsPlot.html.
✖
Wolfram Language. 2010. "NicholsPlot." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2014. https://reference.wolfram.com/language/ref/NicholsPlot.html.APA
Wolfram Language. (2010). NicholsPlot. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/NicholsPlot.html
✖
Wolfram Language. (2010). NicholsPlot. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/NicholsPlot.htmlBibTeX
✖
@misc{reference.wolfram_2025_nicholsplot, author="Wolfram Research", title="{NicholsPlot}", year="2014", howpublished="\url{https://reference.wolfram.com/language/ref/NicholsPlot.html}", note=[Accessed: 24-April-2025
]}BibLaTeX
✖
@online{reference.wolfram_2025_nicholsplot, organization={Wolfram Research}, title={NicholsPlot}, year={2014}, url={https://reference.wolfram.com/language/ref/NicholsPlot.html}, note=[Accessed: 24-April-2025
]}