WOLFRAM

Wolfram Language & System Documentation Center
Wolfram Language Home Page »

FourierCosTransform
✖
FourierCosTransform

Updatedshow changeshide changes

✖
FourierCosTransform[expr,t,ω]

gives the symbolic Fourier cosine transform of expr.

✖
FourierCosTransform[expr,{t1,t2,…},{ω1,ω2,…}]

gives the multidimensional Fourier cosine transform of expr.

Details and Options

  • The Fourier cosine transform is a particular way of viewing the Fourier transform without the need for complex numbers or negative frequencies.
  • Joseph Fourier designed his famous transform using this and the Fourier sine transform, and they are still used in applications like signal processing, statistics and image and video compression.
  • The Fourier cosine transform of the time domain function 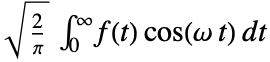is the frequency domain function 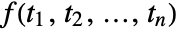for :
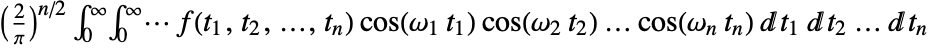  • The Fourier cosine transform of a function is by default defined to be .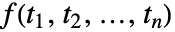
  • The multidimensional Fourier cosine transform of a function 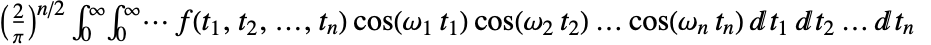is by default defined to be 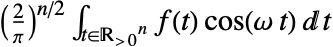or when using vector notation, (2/pi)^(n/2)int_(t in TemplateBox[{}, PositiveReals]^n) f(t) cos(omega t)dt.
  • Different choices of definitions can be specified using the option FourierParameters.
  • The integral is computed using numerical methods if the third argument, , is given a numerical value.
  • The asymptotic Fourier cosine transform can be computed using Asymptotic.
  • There are several related Fourier transformations:
  • FourierTransforminfinite continuous-time functions (FT)
    FourierSequenceTransforminfinite discrete-time functions (DTFT)
    FourierCoefficientfinite continuous-time functions (FS)
    Fourierfinite discrete-time functions (DFT)
  • The Fourier cosine transform is an automorphism in the Schwartz vector space of functions whose derivatives are rapidly decreasing and thus induces an automorphism in its dual: the space of tempered distributions. These include absolutely integrable functions, well-behaved functions of polynomial growth and compactly supported distributions.
  • Hence, FourierCosTransform not only works with absolutely integrable functions on , but it can also handle a variety of tempered distributions such as DiracDelta to enlarge the pool of functions or generalized functions it can effectively transform.
  • The lower limit of the integral is effectively taken to be TemplateBox[{0, -}, Superscript], so that the Fourier cosine transform of the Dirac delta function is equal to . »
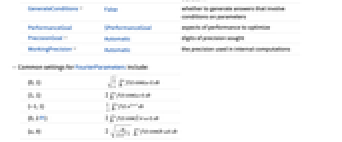
  • The following options can be given:
  • AccuracyGoal Automaticdigits of absolute accuracy sought
    Assumptions $Assumptionsassumptions to make about parameters
    FourierParameters {0,1}parameters to define the Fourier cosine transform
    GenerateConditions Falsewhether to generate answers that involve conditions on parameters
    PerformanceGoal$PerformanceGoalaspects of performance to optimize
    PrecisionGoal Automaticdigits of precision sought
    WorkingPrecision Automaticthe precision used in internal computations
  • Common settings for FourierParameters include:
  • {0,1}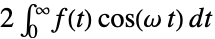
    {1,1}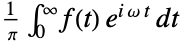
    {-1,1}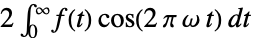
    {0,2Pi}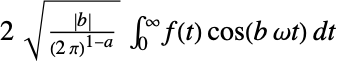
    {a,b}

Examples

open allclose all

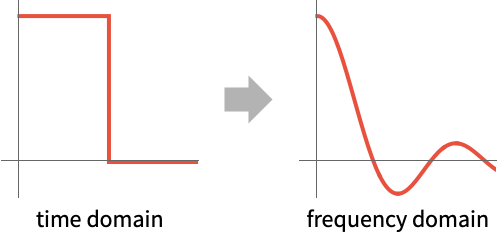
Basic Examples  (6)Summary of the most common use cases

Compute the Fourier cosine transform of a function:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-p2tcor
Out[1]=1

Plot the function and its Fourier cosine transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-6ye72y
Out[2]=2

Fourier cosine transform of reciprocal square root:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-dcw0u5
Out[1]=1

For a different convention, change the parameters:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-qgnjxj
Out[1]=1

Fourier cosine transform of a Gaussian is another Gaussian:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ww5ktw
Out[1]=1

Plot both Gaussians:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-1v7uvx
Out[2]=2

Compute the Fourier cosine transform of a multivariate function:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-thp334
Out[1]=1

Plot the result:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-wzr4fg
Out[2]=2

Compute the transform at a single point:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-dvi4zc
Out[1]=1

Scope  (39)Survey of the scope of standard use cases

Basic Uses  (3)

Fourier cosine transform of a function for a symbolic parameter :

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-5bs32x
Out[1]=1

Fourier cosine transforms involving trigonometric functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-k1cefg
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-esenq3
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-pgpp70
Out[3]=3

Plot the transform for :

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-guxs6c
Out[4]=4

Evaluate the Fourier cosine transform for a numerical value of the parameter :

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-jtuydl
Out[1]=1

Algebraic Functions  (4)

Fourier cosine transform of power functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-gwmclb
Out[1]=1

Cosine transform for rational functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-g56fuq
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-fjkzat
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-gwgokl
Out[3]=3

Plot the transform:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-0zcnkl
Out[4]=4

Fourier cosine transform of a quotient of two nonlinear polynomials:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-550jfl
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-5kvwu
Out[2]=2

Fourier cosine transform of a quotient of quadratic and quartic polynomials:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-69mtza
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-xlrn8r
Out[2]=2

Exponential and Logarithmic Functions  (3)

Fourier cosine transform of an exponential function:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-f07azt
Out[1]=1

Transform for :

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-cedi5l
Out[2]=2

Plot the transform:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-wu1agn
Out[3]=3

Fourier cosine transforms of products of exponential and trigonometric functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-9gu2r1
Out[1]=1

Plot the transform for and :

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-sxteev
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-4zknlr
Out[3]=3

Plot the transform:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-3pnitz
Out[4]=4

Cosine transforms of logarithmic functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-rvogt8
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-3seif6
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ukk5x8
Out[3]=3

Plot the transform:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-taggka
Out[4]=4
In[5]:=5
✖
https://wolfram.com/xid/0mk7q7rt5qb2-7pmgex
Out[5]=5

Plot the transform:

In[6]:=6
✖
https://wolfram.com/xid/0mk7q7rt5qb2-p0d40t
Out[6]=6
In[7]:=7
✖
https://wolfram.com/xid/0mk7q7rt5qb2-2nvqvz
Out[7]=7

Plot the transform:

In[8]:=8
✖
https://wolfram.com/xid/0mk7q7rt5qb2-cv28j0
Out[8]=8

Trigonometric Functions  (5)

Expressions involving trigonometric functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ce36ke
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-cvajrq
Out[2]=2

Composition of elementary functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-1x47i6
Out[1]=1

Plot the transform for :

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-1f40do
Out[2]=2

Ratio of sine and product of exponential and linear functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-g5t4pp
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-xd3bye
Out[2]=2

Fourier cosine transform of arctangent functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-0fdg43
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-y8iia5
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-2n9z25
Out[3]=3

Plot the transform:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-dvz0vq
Out[4]=4

Fourier cosine transform of Sech is another Sech:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-wtwgxh
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-xyvbln
Out[2]=2

Special Functions  (7)

Sinc function:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-31q6j8
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-vo1vn2
Out[2]=2

Fourier cosine transforms of expressions involving ExpIntegralEi:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-6qd4e0
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-34zfcg
Out[2]=2

Expression involving Erfc:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-g7ojs9
Out[1]=1

Plot the transform for :

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-m91upc
Out[2]=2

Expression involving SinIntegral:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-spacqd
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-3gwf7z
Out[2]=2

CosIntegral:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-4ryyap
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-lpmhs9
Out[2]=2

Cosine transforms for BesselJ functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-1dema4
Out[1]=1

Plot the transform for :

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-fzv48
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ujzfxs
Out[3]=3

Plot the transform for and :

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-5ikc3a
Out[4]=4
In[5]:=5
✖
https://wolfram.com/xid/0mk7q7rt5qb2-fota1b
Out[5]=5

Plot the transform for and :

In[6]:=6
✖
https://wolfram.com/xid/0mk7q7rt5qb2-oujib3
Out[6]=6
In[7]:=7
✖
https://wolfram.com/xid/0mk7q7rt5qb2-8916gw
Out[7]=7

Plot the transform:

In[8]:=8
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ucezzb
Out[8]=8

Cosine transforms for BesselY functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-gymguc
Out[1]=1

Plot the transform for :

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-f8qei2
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ksw98u
Out[3]=3

Plot the transform for and :

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-z955xt
Out[4]=4

Piecewise Functions and Distributions  (4)

Fourier cosine transform of a piecewise function:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-wmi67f
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-2fzir2
Out[3]=3

Restriction of a sine function to a half-period:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-8an9nd
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-75reqt
Out[2]=2

Triangular function:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-vtshg5
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-yuhkm5
Out[2]=2

Transforms in terms of FresnelC:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-3vutk1
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-fye5aq
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-u5hicw
Out[3]=3

Plot the transform:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-l8c2cs
Out[4]=4

Periodic Functions  (2)

Fourier cosine transform of cosine:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-i383mn
Out[1]=1

Fourier cosine transform of SquareWave:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-1ay4cq
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-pp7b3q
Out[2]=2

Generalized Functions  (4)

Fourier cosine transforms of expressions involving HeavisideTheta:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-f2e1xq
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-n43u35
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-e43qk9
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-l3p5a2
Out[4]=4

Fourier cosine transforms involving DiracDelta:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-1fgrwd
Out[1]=1

Plot the transform:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-9rmagq
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-bmouhy
Out[3]=3

Plot the transform:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-qtq8ux
Out[4]=4
In[5]:=5
✖
https://wolfram.com/xid/0mk7q7rt5qb2-n4qcpi
Out[5]=5

Plot the transform:

In[6]:=6
✖
https://wolfram.com/xid/0mk7q7rt5qb2-5gk41t
Out[6]=6

Fourier cosine transform involving HeavisideLambda:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-7c77l1
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-xz26mz
Out[2]=2

Fourier cosine transform involving HeavisidePi:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-1gzes
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-owzoy3
Out[2]=2

Multivariate Functions  (2)

Fourier cosine transforms of exponentials in two variables:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-m5wgxc
Out[1]=1

Plot of both:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-8dlol1
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-t352wi
Out[3]=3

Plot of both:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-5xlxxb
Out[4]=4

Fourier cosine transform of product of exponential and SquareWave:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-8haesz
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-u1nlix
Out[2]=2

Formal Properties  (3)

Fourier cosine transform of a first-order derivative:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-qh1s7g
Out[1]=1

Fourier cosine transform of a second-order derivative:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-v762xl
Out[1]=1

Fourier cosine transform threads itself over equations:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-8u929y
Out[1]=1

Numerical Evaluation  (2)

Calculate the Fourier cosine transform at a single point:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-b0w5t
Out[1]=1

Alternatively, calculate the Fourier cosine transform symbolically:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-nz33l8
Out[1]=1

Then evaluate it for specific value of :

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-j4vp9p
Out[2]=2

Options  (8)Common values & functionality for each option

AccuracyGoal  (1)

The option AccuracyGoal sets the number of digits of accuracy:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-5ymjb
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-f6u3ua
Out[2]=2

With default settings:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ld9md4
Out[3]=3

Assumptions  (1)

Fourier cosine transform of BesselJ is a piecewise function:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-nagwk1
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-fk9tc3
Out[2]=2

FourierParameters  (3)

Fourier cosine transform for the unit box function with different parameters:

Use a nondefault setting for a different definition of transform:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ejscab
Out[1]=1

To get the inverse, use the same FourierParameters setting:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-j5l0np
Out[2]=2

Set up your particular global choice of parameters once per session:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-82g0uf
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-gz54fq

Restore defaults:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-8p4bec
Out[3]=3

GenerateConditions  (1)

Use GenerateConditionsTrue to get parameter conditions for when a result is valid:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-dosv41
Out[1]=1

PrecisionGoal  (1)

The option PrecisionGoal sets the relative tolerance in the integration:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-n3x5d0
Out[1]=1
In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-9119x
Out[4]=4

With default settings:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-b3dg8e
Out[3]=3

WorkingPrecision  (1)

If a WorkingPrecision is specified, the computation is done at that working precision:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-dipupy
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-duvdjv
Out[2]=2

With default settings:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-iorkcp
Out[3]=3

Applications  (4)Sample problems that can be solved with this function

Ordinary Differential Equations  (1)

Consider the following ODE with initial condition :

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-7nyko5
Out[1]=1

Apply the Fourier cosine transform to the ODE:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-vneovh
Out[2]=2

Solve for the Fourier cosine transform of :

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-o8i7eo
Out[3]=3

Find the inverse Fourier cosine transform with and :

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-o2vnsc
Out[4]=4

Compare with DSolveValue:

In[5]:=5
✖
https://wolfram.com/xid/0mk7q7rt5qb2-p6a0xe
Out[5]=5

Partial Differential Equations  (1)

Solve the heat equation for , : with initial condition for and Neumann boundary condition for .

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-px64a0

Apply the Fourier cosine transform to the ODE on :

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-2kme07
Out[2]=2

With and , solve this ODE:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ehtvs7
Out[3]=3

Compute the inverse cosine transform of the exponential functions:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ccx48j

The convolution property gives the inverse cosine transform of the first summand to get the solution:

In[6]:=6
✖
https://wolfram.com/xid/0mk7q7rt5qb2-h6hd7
Out[6]=6

Consider the special case with , and :

In[7]:=7
✖
https://wolfram.com/xid/0mk7q7rt5qb2-jgjd13
Out[7]=7

Compare with DSolveValue:

In[8]:=8
✖
https://wolfram.com/xid/0mk7q7rt5qb2-dkim4w
Out[8]=8

Plot the initial conditions and solutions for different values of .

In[9]:=9
✖
https://wolfram.com/xid/0mk7q7rt5qb2-t3wb9i
Out[9]=9

Evaluation of Integrals  (2)

Calculate the following definite integral:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-mvl6f4
Out[1]=1

Fourier cosine transform preserves integration of products over :

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-0i61ex
Out[2]=2

Solve the definite integral:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-gojuf4
Out[3]=3

Compare with Integrate:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-8yvy61
Out[4]=4

Calculate the following definite integral for :

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-xpx1az
Out[1]=1

Compute Fourier cosine transform of an exponential function:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-g76rkm
Out[2]=2

Apply Parseval's identity:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-l3jwfg
Out[3]=3

Or equivalently:

In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-5w5yeo
Out[4]=4

Solve for the definite integral:

In[5]:=5
✖
https://wolfram.com/xid/0mk7q7rt5qb2-y2ew8h
Out[5]=5

Compare with Integrate:

In[6]:=6
✖
https://wolfram.com/xid/0mk7q7rt5qb2-e84l8t
Out[6]=6

Properties & Relations  (4)Properties of the function, and connections to other functions

By default, the Fourier cosine transform of is:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-nbvwzu

For , the definite integral becomes:

In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-tj5dfk
Out[2]=2

Compare with FourierCosTransform:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-oy2spl
Out[3]=3

Use Asymptotic to compute an asymptotic approximation:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-lwbzos
Out[1]=1

FourierCosTransform and InverseFourierCosTransform are mutual inverses:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-bp78fy
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-bhj9ka
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-it7bfn
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0mk7q7rt5qb2-byd6ra
Out[4]=4

Results from FourierCosTransform and FourierTransform agree for even functions:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-coouyp
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-dn4tm5
Out[2]=2

The results agree for :

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-b2ebmr
Out[3]=3

Possible Issues  (1)Common pitfalls and unexpected behavior

The result from an inverse Fourier cosine transform may not have the same form as the original:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-e33562
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-0996p9
Out[2]=2

Fourier cosine transform may be given in terms of generalized functions such as DiracDelta:

In[3]:=3
✖
https://wolfram.com/xid/0mk7q7rt5qb2-mjrqa7
Out[3]=3

Neat Examples  (2)Surprising or curious use cases

Fourier cosine transform as a Meijer function:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-ebx4b0
Out[1]=1

Create a table of basic Fourier cosine transforms:

In[1]:=1
✖
https://wolfram.com/xid/0mk7q7rt5qb2-p2zt94
In[2]:=2
✖
https://wolfram.com/xid/0mk7q7rt5qb2-3e19ls
Wolfram Research (1999), FourierCosTransform, Wolfram Language function, https://reference.wolfram.com/language/ref/FourierCosTransform.html (updated 2025).
✖
Wolfram Research (1999), FourierCosTransform, Wolfram Language function, https://reference.wolfram.com/language/ref/FourierCosTransform.html (updated 2025).

Text

Wolfram Research (1999), FourierCosTransform, Wolfram Language function, https://reference.wolfram.com/language/ref/FourierCosTransform.html (updated 2025).

✖
Wolfram Research (1999), FourierCosTransform, Wolfram Language function, https://reference.wolfram.com/language/ref/FourierCosTransform.html (updated 2025).

CMS

Wolfram Language. 1999. "FourierCosTransform." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2025. https://reference.wolfram.com/language/ref/FourierCosTransform.html.

✖
Wolfram Language. 1999. "FourierCosTransform." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2025. https://reference.wolfram.com/language/ref/FourierCosTransform.html.

APA

Wolfram Language. (1999). FourierCosTransform. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/FourierCosTransform.html

✖
Wolfram Language. (1999). FourierCosTransform. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/FourierCosTransform.html

BibTeX

@misc{reference.wolfram_2025_fouriercostransform, author="Wolfram Research", title="{FourierCosTransform}", year="2025", howpublished="\url{https://reference.wolfram.com/language/ref/FourierCosTransform.html}", note=[Accessed: 20-April-2025 ]}

✖
@misc{reference.wolfram_2025_fouriercostransform, author="Wolfram Research", title="{FourierCosTransform}", year="2025", howpublished="\url{https://reference.wolfram.com/language/ref/FourierCosTransform.html}", note=[Accessed: 20-April-2025 ]}

BibLaTeX

@online{reference.wolfram_2025_fouriercostransform, organization={Wolfram Research}, title={FourierCosTransform}, year={2025}, url={https://reference.wolfram.com/language/ref/FourierCosTransform.html}, note=[Accessed: 20-April-2025 ]}

✖
@online{reference.wolfram_2025_fouriercostransform, organization={Wolfram Research}, title={FourierCosTransform}, year={2025}, url={https://reference.wolfram.com/language/ref/FourierCosTransform.html}, note=[Accessed: 20-April-2025 ]}